NCERT Solutions for Class 11 Chemistry Chapter 5 States of Matter

Access premium articles, webinars, resources to make the best decisions for career, course, exams, scholarships, study abroad and much more with

Plan, Prepare & Make the Best Career Choices

NCERT Solutions for Class 11 Chemistry Chapter 5 States of Matter

Edited By Sumit Saini | Updated on Aug 18, 2022 04:03 PM IST

NCERT solutions for class 11 chemistry chapter 5 States of Matter- The matter is existing in 3 physical states which are solid, liquid and gas. In NCERT solutions for class 11 chemistry chapter 5 States of matter, you will deal with the two states of matter namely liquid state and gaseous state, the laws governing the behaviour of ideal gases and properties associated with liquids. In NCERT syllabus class 11 chemistry chapter 5 States of Matter, there are 23 questions in the exercise.

JEE Main Scholarship Test Kit (Class 11): Narayana | Physics Wallah | Aakash | Unacademy

NEET Scholarship Test Kit (Class 11): Narayana | Physics Wallah | Aakash | ALLEN

Suggested: JEE Main: high scoring chapters | Past 10 year's papers

New: Aakash iACST Scholarship Test. Up to 90% Scholarship. Register Now

Also Read :

The NCERT solutions for class 11 chemistry chapter 5 States of Matter are prepared and solved by subject experts. These NCERT solutions will help you in preparation of class 11 final examination as well as in the preparation of various competitive exams like NEET, JEE, BITSAT etc. By referring to the NCERT solutions for class 11 , students can understand all the important concepts and practice questions well enough before their examination.

Some common characteristics of three forms of matter solid liquid and gas are summarised below-

S.No.	Gases	Liquids	Solids
1	No definite shape	No definite shape	Definite shape
2	Indefinite volume	Definite volume	Definite volume
3	Compressible	Slightly compressible	Nearly compressible
4	Low density	Intermediate density	High density

NCERT Solutions for Class 11 Chemistry Chapter 5 States of Matter- Exercise Questions

Question 5.1 What will be the minimum pressure required to compress $500\; dm^{3}$ of air at 1 bar to $200\; dm^{3}$ at $30^{o}C$ ?

Answer :

Given the volume of air to be compressed is $500dm^3$ at $1 bar$ pressure to $200dm^3$ at $30^{\circ}C$ .

So, as the temperature remains constant at $30^{\circ}C$ .

We have Boyle's law where, $P_{1}V_{1} = P_{2}V_{2}$

To calculate the final pressure $P_{2}$ .

We have,

$P_{1}V_{1} = P_{2}V_{2}$

$P_{2} = \frac{P_{1}V_{1}}{V_{2}}$

$= \frac{1\times 500}{200} bar = 2.5\ bar$

Therefore, the minimum pressure required is $2.5\ bar$ .

Question 5.2 A vessel of $120 \; mL$ capacity contains a certain amount of gas at $35^{o}C$ and 1.2 bar pressure. The gas is transferred to another vessel of volume $180 \; mL$ at $35^{o}C$ . What would be its pressure?

Answer :

Given volume of air to be transferred from the capacity of $120 \; mL$ vessel at $1.2\ bar$ pressure to $180 \; mL$ vessel at $35^{o}C$

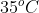
So, as the temperature remains constant at 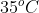$35^{o}C$ .

We have Boyle's law where, $P_{1}V_{1} = P_{2}V_{2}$

To calculate the final pressure $P_{2}$ .

We have,

$P_{1}V_{1} = P_{2}V_{2}$

$P_{2} = \frac{P_{1}V_{1}}{V_{2}}$

$= \frac{1.2\times 120}{180} bar = 0.8\ bar$

Therefore, the pressure required is $0.8\ bar$ .

Question 5.3 Using the equation of state $pV=nRT;$ show that at a given temperature density of a gas is proportional to gas pressure p.

Answer :

Given ideal gas equation ;

$PV = nRT$

So, at given fixed temperature T. we have the

$Density\ of\ gas (\rho ) = \frac{mass(m)}{Volume(V)}$

And we know $n = \frac{Mass\ of\ gas}{Molar\ mass\ of\ gas}$

so, we get $P = \frac{mRT}{MV}$

$P = \frac{\rho RT}{M}$

But at constant Temperature, we have $P \propto \rho$ .

Question 5.4 At $0^{o}\; C$ , the density of a certain oxide of a gas at 2 bar is same as that of dinitrogen at 5 bar. What is the molecular mass of the oxide?

Answer :

Given the condition :

Temperature $= 0^{\circ}C$ ,

$Pressure = 2\ bar$ for oxide gas and $Pressure = 5\ bar$ for dinitrogen.

As Density of gas is represented by, $d = \frac{PM}{RT}$

And given that density is the same for both the gases at the same temperature condition.

So, we have $M_{1}P_{1} = M_{2}P_{2}$ (as R is constant}

or, $M_{1}\times 2 =28\times 5$ $(\because Molecular\ mass\ of\ N_2 = 28\ u)$

or, $M_{1} = 70\ u$

Question 5.5 Pressure of 1 g of an ideal gas A at $27^{o}C$ is found to be 2 bar. When 2 g of another ideal gas B is introduced in the same flask at the same temperature the pressure becomes 3 bar. Find a relationship between their molecular masses.

Answer :

Given Pressure of 1g of an ideal gas A at $27^{\circ}C$ is $P_{A} = 2\ bar$ .

When 2g of another ideal gas B is introduced in the same flask at the same temperature,

The pressure becomes $P_{A}+P_{B}= 3\ bar$ .

$\implies P_{B }= 1\ bar$ .

We can assume the molecular masses of A and B be $M_{A}\ and\ M_{B}$ respectively.

The ideal gas equation, $PV=nRT$

So, we have $P_{A}V=n_{A}RT$ and $P_{B}V=n_{B}RT$

Therefore, $\frac{P_{A}}{P_{B}} = \frac{n_{A}}{n_{B}} = \frac{1M_{B}}{2M_{A}} = \frac{M_{B}}{2M_{A}}$

or, $\frac{M_{B}}{M_{A}} = 2\times\frac{P_{A}}{P_{B}} = 2\times \frac{2}{1} =4$

Hence the relation between the two gases is $M_{B} =4M_{A}$ .

Question 5.6 The drain cleaner, Drainex contains small bits of aluminum which react with caustic soda to produce dihydrogen. What volume of dihydrogen at 20 °C and one bar will be released when 0.15g of aluminum reacts?

Answer :

We have the chemical reaction:

$2 Al +2NaOH +H_2O \rightarrow 2NaAlO_2+3H_{2}$

(Where dihydrogen is being produced.)

So, at $20^{\circ}C$ and $1\ bar$ pressure, the volume of gas that will be released when $0.15g$ of aluminium reacts.

As we can see from the reaction equation that 2 moles of aluminium produce 3 moles of dihydrogen.

i.e., $2\times 27g$ reacts to give $3\times 22.4Litres$

At STP condition

$273.15K$ and $1atm$ ,

$54g(2\times 27g)$ of Al gives $3\times 22400mL$ of $H_{2}$ .

Therefore $0.15g$ of Al will give $= \frac{3\times22400\times0.15}{54}mL$ of $H_{2}$

i.e., $186.67mL$ of $H_{2}$ .

At STP condition:

$273.15K$ and $1atm$ ,

Now, $P_{1} =1atm$ , $V_{1} = 186.67mL$ , $T_{1} = 273.15K$

Then we assume the volume of dihydrogen be $V_{2}$ at the pressure $P_{2} = 0.987atm$

(Since 1 bar = 0.987atm) and temperature $T_{2} = 20^{\circ}C$

$\implies (273.15+20)K = 293.15K$

$\frac{P_{1}V_{1}}{T_{1}} = \frac{P_{2}V_{2}}{T_{2}}$

$V_{2}= \frac{P_{1}V_{1}T_{2}}{P_{2}T_{1}}$

$= \frac{1\times186.67\times293.15}{0.987\times273.15}$

$= 202.98mL\approx 203mL$

Therefore, 203mL of dihydrogen will be released.

Question 5.7 What will be the pressure exerted by a mixture of 3.2 g of methane and 4.4 g of carbon dioxide contained in a $9\; dm^{3}$ flask at $27 ^{o}C$ ?

Answer :

Given a mixture of 3.2g of methane and 4.4g of carbon dioxide contained a $9dm^3$ flask at $27 ^{o}C$ .

So, the pressure exerted by the mixture will be

$P =\frac{n}{V}RT = \frac{m}{M}\frac{RT}{V}$

The pressure exerted by the Methane gas, $P_{CH_{4}} =(\frac{3.2mol}{16}) \frac{0.0821 dm^3 atm K^{-1}mol^{-1}\times300K}{9 dm^3} = 0.55\ atm$

The pressure exerted by the Carbon dioxide gas,

$P_{CO_{2}} =(\frac{4.4mol}{44}) \frac{0.0821 dm^3 atm K^{-1}mol^{-1}\times300K}{9 dm^3} = 0.27\ atm$

So, the total pressure exerted $= 0.55+0.27 = 0.82\ atm$

And in terms of SI units, we get,

$R = 8.314p\ m^3\ K^{-1}mol^{-1},\ V= 9\times 10^{-3}\ m^3$

$P = 5.543\times10^4 Pa +2.771\times 10^4Pa = 8.314\times10^4 Pa.$

Question 5.8 What will be the pressure of the gaseous mixture when $0.5\: L$ of $H_{2}$ at $0.8$ bar and $2.0\: L$ of dioxygen at $0.7$ bar are introduced in a 1L vessel at $27^{o}C$ ?

Answer :

Pressure of the gas mixture will be the sum of partial pressure of $H_{2}$ gas and partial pressure of $O_{2}$ gas.

So, calculating the partial pressures of each gas.

Partial pressure of $H_{2}$ in a 1L volume vessel.

$P_{1} = 0.8\ bar$ , $P_{2},\ V_{1} = 0.5L,\ V_{2} =1L$

As the temperature remains constant, $P_{1}V_{1} =P_{2}V_{2}$

$\implies (0.8\ bar)(0.5L) = P_{2}(1L)$

or $P_{2} = 0.4\ bar$ or $P_{H_{2}} = 0.4\ bar$

Now, calculating the partial pressure of $O_{2}$ gas in 1L vessel.

$P'_{1}V_{1} = P'_{2} V'_{2}$

$\implies (0.7\ bar)(2L) = P_{2}(1L)$

$\implies P'_{2} = 1.4\ bar$ or $P_{O_{2}} = 1.4\ bar$

Therefore the total pressure $= P_{H_{2}} +P_{O_{2}} = 0.4\ bar+1.4\ bar = 1.8\ bar$

Question 5.9 Density of a gas is found to be $5.46 \: g/dm^{3}$ at $27^{o}C$ at 2 bar pressure. What will be its density at STP ?

Answer :

Given the density of a gas is equal to $5.46g/dm^3$ at $27^{\circ}C$ and at 2bar pressure.

Density $d = \frac{PM}{RT}$ , for the same gas at different temperatures and pressures we can apply,

$\frac{d_{1}}{d_{2}} = \frac{P_{1}}{T_{1}}\times\frac{T_{2}}{P_{2}}.$

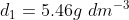Here, $d_{1} =5.46g\ dm^{-3}$ , $T_{1} =27^{\circ}C =300K$ , $P_{1} =2bar.$

then at STP, we have $d_{2},\ T_{2} = 0^{\circ}C =273K,\ P_{2} =1bar.$

$\therefore \frac{5.46g\ dm^{-3}}{d_{2}} = \frac{2bar}{300K}\times\frac{273K}{1bar}$ or $d_{2} = 3g\ dm^{-3}$

Question 5.10 $34.05 \; mL$ of phosphorus vapour weighs 0.0625 g at $546 ^{o}C$ and 0.1 bar pressure. What is the molar mass of phosphorus?

Answer :

Given,

The volume of phosphorus vapour $= 34.05mL$ which weights about $0.0625g$ .

Temperature $= 546^{\circ}C = 273+546 = 819K$

Pressure $=0.1 bar$

Hence we apply the ideal gas equation,

$PV=nRT$ , where the number of moles will be $n =\frac{PV}{RT}$

$= \frac{1bar\times (34.05\times 10^{-3} dm^3)}{0.083 bar\ dm^3\ K^{-1}Mol^{-1}\times 819K}$

$= 5\times 10^{-4} mol$

$\therefore Mass\ of\ 1\ mole = \frac{0.0625}{5\times 10^{-4}}g = 125g$

Therefore the molar mass is $125g\ mol^{-1}$

Question 5.11 A student forgot to add the reaction mixture to the round bottomed flask at 27 °C but instead he/she placed the flask on the flame. After a lapse of time, he realized his mistake, and using a pyrometer he found the temperature of the flask was 477 °C. What fraction of air would have been expelled out?

Answer :

Assume the round-bottomed flask has volume $= V\ cm^3$

so, the volume of air in the flask at $27^{\circ}C$ $= V\ cm^3$ .

To find out how much air has been expelled out, we use Charle's Law:

$\frac{V_{1}}{T_{1}} = \frac{V_{2}}{T_{2}}$

Therefore $Volume\ expelled = 2.5V-V =1.5V$

Or, Fraction of air expelled $=\frac{1.5V}{2.5V} =\frac{3}{5}$

Question 5.12 Calculate the temperature of $4.0 \: mol$ of a gas occupying $5\: \: dm^{3}$ at 3.32 bar.
$(R = 0.083 \: bar\: dm^{3} K^{-1} mol^{-1}).$

Answer :

We have the ideal gas equation,

$PV=nRT$

Pressure, p=3.32bar

Volume, V=5 $dm^3$

Number of moles, n=4 mol

Gas constant R= 0.083

Temperature, T=?

$T = \frac{PV}{nR} = \frac{3.32bar\times 5\ dm^3}{4\ mol\times 0.083 bar\ dm^3\ K^{-1}mol^{-1}} = 50K$

so, temperature, T=50K

Question 5.13 Calculate the total number of electrons present in 1.4 g of dinitrogen gas.

Answer :

Dinitrogen $N_{2}$ has a molar mass $= 28g\ mol^{-1}$ .

Given 1.4g of $N_{2}$ gas.

That means $\frac{1.4}{28} = 0.05\ mol$

$= 0.05\times6.02\times10^{23} = 3.01\times 10^{23}$ number of molecules.

And as 1 molecule of $N_{2}$ has 14 electrons.

So, $3.01\times 10^{23}$ molecules of $N_{2}$ has $14\times 3.01\times 1023 = 4.214\times 10^{23}$ electrons.

Question 5.14 How much time would it take to distribute one Avogadro number of wheat grains, if 1010 grains are distributed each second ?

Answer :

Given that $10^{10}$ grains are distributed each second.

Time taken to distribute $10^{10}$ grains = 1second.

Time taken to distribute one Avogadro number of wheat grains.

$=\frac{1s\times 6.022\times 10^{23}grains}{10^{10}grains}$

$= \frac{6.022\times10^{23}}{60\times 60\times 24\times 365} = 1.9\times 10^{6}\ years.$

Question 5.15 Calculate the total pressure in a mixture of 8 g of dioxygen and 4 g of dihydrogen confined in a vessel of $1\: dm^{3}$ at 27°C. $R = 0.083\: bar \: dm^{3} K^{-1} mol^{-1}$ .

Answer :

Given the mass of oxygen gas and mass of hydrogen gas.

Molar mass of $O_{2} =32g\ mol^{-1}$

Therefore $8g\ O_{2} = \frac{8}{32}mol = 0.25\ mol$

Molar mass of $H_{2} = 2g\ mol^{-1}$

Therefore $4g H_{2} =\frac{4}{2} =2\ mol$

$\Rightarrow$ Total number of moles of mixture $n = 2+0.25 =2.25$

and $V = 1\ dm^3,\ T = 27^{\circ }C = 300K,\ R=0.083\ bar\ dm^{3}K^{-1}\ mol^{-1}$

So, Ideal gas equation; $PV = nRT$

or $P = \frac{nRT}{V} = \frac{(2.25mol\times) (0.083 bar\ dm^3K^{-1}mol^{-1})(300K)}{1 dm^3}$

Therefore Total pressure is $= 56.025\ bar$

Question 5.16 Pay load is defined as the difference between the mass of displaced air and the mass of the balloon. Calculate the pay load when a balloon of radius 10 m, mass 100 kg is filled with helium at 1.66 bar at 27°C.

(Density of $air = 1.2 \: kg\: m^{-3}$ and $R = 0.083 \: bar \: dm^{3} K^{-1} mol^{-1}$ ).

Answer :

The payload can be defined as:

(Mass of the displaced air - Mass of the balloon)

Given the radius of the balloon, r = 10 m.

Mass of the balloon, m = 100 kg.

Therefore, the volume of the balloon will be:

$V = \frac{4}{3}\pi r^3 = \frac{4}{3}\times\frac{22}{7}\times10^3 = 4190.5\ m^3$

Now, the volume of the air displaced:

$V_{d} = 4190.5\ m^3$

The mass of the air displaced :

$m_{d} = density \times V_{d} = 1.2kgm^{-3}\times 4190.5\ m^3$

$= 5028.6kg$

Let $W$ be the mass of helium gas filled into the balloon, then

$PV=(\frac{W}{m})rt$

Or, $W = \frac{PVM}{RT}$

$= \frac{1.66\times4190.5\times10^3\times4}{0.083\times300} = 1117\ kg$ approximately.

The balloon is filled with He with total mass of $= 1117+100= 1217kg$

$\therefore$ The payload of the balloon will be:

$= 5028.6 -1217 = 3811.6kg$

Question 5.17 Calculate the volume occupied by 8.8 g of $CO_{2}$ at 31.1°C and 1 bar pressure. $R = 0.083 \: bar \: L\: K^{-1} mol^{-1}$ .

Answer :

Given the mass of carbon dioxide is 8.8grams at $31.1^{\circ}C$ and at 1 bar pressure.

So, the number of moles of

$CO_{2} (n) = \frac{Mass\ of\ CO_{2}}{Molar\ Mass}$

$= \frac{8.8g}{44g\ mol^{-1}} = 0.2\ mol$

Now, the pressure of $CO_{2} (P) = 1\ bar$

Given $R = 0.083 \: bar \: L\: K^{-1} mol^{-1}$

also, the $Temperature (T) = 273+31.1 = 304.1K$

The Ideal gas equation; $PV=nRT$

$V=\frac{nRT}{P} = \frac{0.2\times 0.083\times304.1}{1\ bar} = 5.048L$

So volume occupied by 8.8 g of carbon dioxide is 5.048L

Question 5.18 2.9 g of a gas at 95 °C occupied the same volume as 0.184 g of dihydrogen at 17 °C, at the same pressure. What is the molar mass of the gas?

Answer :

Let the molar mass of the gas be $M_{gas}$ ,

Then, given that $2.9g$ of a gas at $95^{\circ}C$ occupied the same volume as

$0.184g$ of $H_{2}$ at $17^{\circ }C$

So, we have the relation, $P_{1}=P_{2}$ and $V_{1} = V_{2}$

Therefore $P_{1}V_{2} = P_{2} V_{2}$

wihch gives, $n_{1}RT_{1} =n_{2}RT_{2}$

$\implies n_{1}T_{1} =n_{2}T_{2}$

Or, $\frac{2.9}{M_{gas}}\times (95+273) = \frac{0.184}{2}\times(17+273)$

Or, $M_{gas} = \frac{2.9\times368\times 2 }{0.184\times 290} = 40g\ mol^{-1}$

Question 5.19 A mixture of dihydrogen and dioxygen at one bar pressure contains 20% by weight of dihydrogen. Calculate the partial pressure of dihydrogen.

Answer :

A mixture of dihydrogen and dioxygen at one bar pressure contains 20% by weight of dihydrogen.

So, let us assume the weight of dihydrogen in a total mixture weight of $W$ be $20Wgrams$ and dioxygen be $80Wgrams$ .

Then, the number of moles of $H_{2}$ will be, $n_{H_{2}} = \frac{20W}{2} = 10W\ moles$ and the number of moles of $O_{2}$ , $n_{O_{2}} = \frac{80W}{32} = 2.5W\ moles$ .

And given that the total pressure of the mixture is $P_{Total} = 1\ bar$ .

Then we have a partial pressure of $H_{2}$ ,

$P_{H_{2}}=(Mol.\ fraction)_{H_{2}}\times P_{Total}$

$= \frac{n_{H_{2}}}{n_{H_{2}}+n_{O_{2}}}\times P_{Total}$

$= \frac{10W}{10W+2.5W}\times 1\ bar$

$=0.8\ bar$

Therefore, the partial pressure of $H_{2}$ is $0.8\ bar$ .

Question 5.20 What would be the $SI$ unit for the quantity $pV^{2}T^{2}/n$ ?

Answer :

Given quantity, $pV^{2}T^{2}/n$ ,

The SI units of each factors are,

For pressure p is $Nm^{-2}$ .

For Volume V is $m^3$ .

For Temperaute T is $K$ .

For number of moles, n is mol.

Therefore we have for the quantity

The SI unit is $=\frac{(Nm^{-2})(m^3)^2)(K)^2}{mol}$

$= Nm^4K^2 mol^{-1}$

Question 5.21 In terms of Charles’ law explain why –273 °C is the lowest possible temperature.

Answer :

Charles' Law states that pressure remaining constant, the volume of a fixed mass of a gas is directly proportional to its absolute temperature.

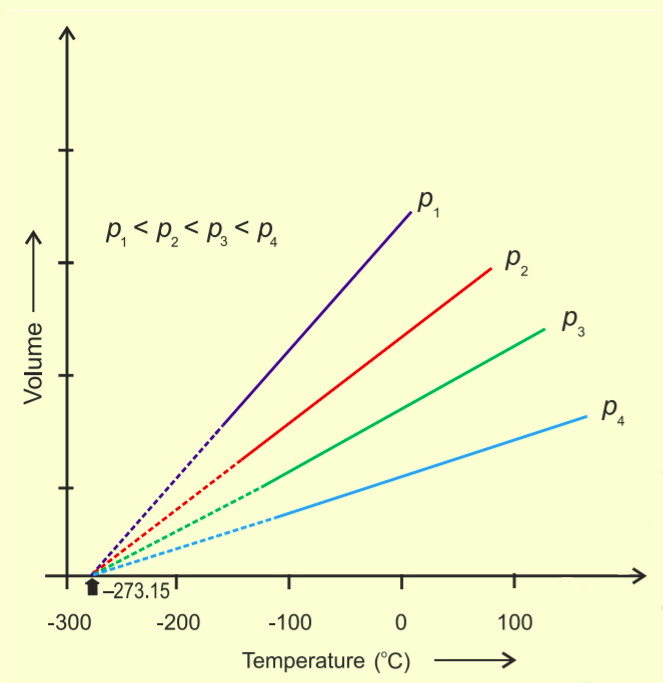
1650521155405

Charles found that for all gases, at any given pressure, the graph of volume vs temperature (in Celsius) is a straight line and on extending to zero volume, each line intercepts the temperature axis at – 273.15 ° C.

We can see that the volume of the gas at – 273.15 ° C will be zero. This means that gas will not exist. In fact, all the gases get liquified before this temperature is reached.

Question 5.22 Critical temperature for carbon dioxide and methane are 31.1 °C and –81.9 °C respectively. Which of these has stronger intermolecular forces and why?

Answer :

The critical temperature we know is the highest temperature at which liquid exists above it is gas.

Given that the critical temperature of Carbon dioxide and Methane are. $31.1 ^{\circ}C\ and\ -81.9^{\circ}C$

Higher is the critical temperature of a gas, easier is its liquefaction.

So, as the critical temperature increases the gas is now easier to liquefaction.

That means the intermolecular forces of attraction between the molecules of a gas are directly proportional to its Critical temperature.

Hence, Carbon dioxide has stronger intermolecular forces than Methane.

Question 5.23 Explain the physical significance of van der Waals parameters.

Answer :

The equation of van der Waals after taking into account the corrections for pressure and volume,

$\left ( p+\frac{an^2}{V^2} \right )\left ( V-nb \right ) =nRT$

Where a and b are called van der Waals constants or parameters.

Here the significance of a and b is important:

Value of ' a' is a measure of the magnitude of intermolecular attractive forces within the gas and is independent of temperature and pressure.

And the value of 'b' is the volume occupied by the molecule and 'nb' is the total volume occupied by the molecules.

More About NCERT Solutions For States Of Matter Class 11 Chemistry

NCERT solutions for class 11 chemistry chapter 5 States of Matter is an important chapter for class 11 students because the topics of this chapter are the basics to the topics to be studied in class 12 NCERT book. The important topics of this chapter are intermolecular forces, thermal energy, the gaseous state, ideal gas equation, kinetic molecular theory of gases, liquid State and more. After completing the NCERT solutions for class 11 chemistry chapter 5 states of matter students will be able to explain the existence of different states of matter, explain the laws governing the behaviour of ideal gases; able to apply gas laws in real life situations; describe the conditions required for liquefaction of gases and also able to differentiate between vapours and gaseous state.

NCERT Solutions for Class 11 Chemistry

Chapter 1	Some Basic Concepts of Chemistry
Chapter-2	Structure of Atom
Chapter-3	Classification of Elements and Periodicity in Properties
Chapter-4	Chemical Bonding and Molecular Structure
Chapter-5	States of Matter
Chapter-6	Thermodynamics
Chapter-7	Equilibrium
Chapter-8	Redox Reaction
Chapter-9	Hydrogen
Chapter-10	The S-Block Elements
Chapter-11	The P-Block Elements
Chapter-12	Organic chemistry- some basic principles and techniques
Chapter-13	Hydrocarbons
Chapter-14	Environmental Chemistry

NCERT Solutions for Class 11 Subject Wise

NCERT solutions for Class 11 Biology

NCERT Solutions for Class 11 Maths

NCERT solutions for Class 11 Chemistry

NCERT solutions for Class 11 Physics

JEE Main Highest Scoring Chapters & Topics

Just Study 40% Syllabus and Score upto 100%

Download EBook

Benefits of NCERT Solutions for Class 11 Chemistry Chapter 5 States of Matter

The solutions are written in a comprehensive manner in the NCERT solutions for class 11 chemistry chapter 5 States of Matter will help you writing answers in your exam.
Revision will be easy because the detailed solutions will help you to remember the concepts and get you good marks.
Homework problems will be easier for you, all you need to do is check the detailed NCERT solutions for class 11 chemistry and you are ready to go.

If you have a doubt or question that is not available here or in any of the chapters, contact us. You will get all the answers that will help you score well in your exams.

Also Check NCERT Books and NCERT Syllabus here:

Frequently Asked Question (FAQs)

1. What is the weightage of states of matter in JEE Mains

This chapter holds weightage of 7 marks in JEE mains

2. Where can I find complete solutions of NCERT class 11 Chemistry

Refer to this link: https://school.careers360.com/ncert/ncert-solutions-class-11-chemistry

3. How are the ncert solutions beneficial for board exam

Most of the questions are asked directly from NCERT, hence it is must to do ncert solutions

4. Whether the unit states of matter is helpful in higher studies?

Yes, it is helpful to a large extent

5. What is the weightage of the chapter states of matter for CBSE board exam

5 marks

6. What is the weightage of NCERT chapter states of matter for NEET exam

This chapter holds weightage of 2%

Also Read

NCERT Class 11 Chemistry Chapter 4 Notes Chemical Bonding and Molecular Structure - Download PDF

NCERT Class 11 Chemistry Chapter 3 Notes - Download PDF

NCERT Class 11 Biology Chapter 19 Notes Excretory Products And Their Elimination- Download PDF Notes

NCERT Class 11 Biology Chapter 22 Notes Chemical Coordination And Integration- Download PDF Notes

Some basic concepts of Chemistry Class 11th Notes - Free NCERT Class 11 Chemistry Chapter 1 Notes - Download PDF

NCERT Class 11 Biology Chapter 21 Notes Neural Control And Coordination- Download PDF Notes

NCERT Books for class 11 Hindi 2024 - Download Free PDF

NCERT Book for class 11 Biology 2024 - Download Free PDF

NCERT Books for class 11 Chemistry 2024 - Download Free Pdf

NCERT Books for class 11 Maths 2024 - Download Free PDF

NCERT Books for Class 11 Physics 2024 - Download PDF

NCERT Books for Class 11 English 2024 - Download Free PDF

NCERT Syllabus for Class 11 2024 - Download All Subjects Pdf Here

NCERT Syllabus for Class 11 English 2024 - Download PDF here

NCERT Class 11 Physics Chapter 5 Notes Laws of Motion - Download PDF

NCERT Class 11 Physics Chapter 3 Notes Motion in a straight Line - Download PDF

NCERT Solutions for Exercise 10.1 Class 11 Maths Chapter 10 - Straight Lines

NCERT Solutions for Miscellaneous Exercise Chapter 16 Class 11 - Probability

NCERT Solutions for Miscellaneous Exercise Chapter 13 Class 11 - Limits and Derivatives

NCERT Solutions for Miscellaneous Exercise Chapter 11 Class 11 - Conic Section

NCERT Exemplar Class 11 Maths Solutions Chapter 12 Introduction to Three Dimensional Geometry

NCERT Exemplar Class 11 Maths Solutions Chapter 10 Straight Lines

NCERT Exemplar Class 11 Maths Solutions Chapter 6 Linear Inequalities

NCERT Exemplar Class 11 Maths Solutions Chapter 16 Probability

NCERT Exemplar Class 11 Maths Solutions Chapter 14 Mathematical Reasoning

NCERT Exemplar Class 11 Maths Solutions Chapter 7 Permutations and Combinations

Articles

Diploma in Stenography - Duration, Eligibility, Top Colleges, Fees

Apr 18, 2024

UP Madarsa Board Result 2024 Out for Molvi, Munshi, Alim, Kamil & Fazil - UPBME Result Here

Apr 17, 2024

Best Lab Technician Courses After 12th: Eligibility, Admission 2024, Colleges, Duration, Fees, Scope

Apr 16, 2024

Graphic Designing Courses after 12th - Eligibility & Top Institutes

Apr 03, 2024

Navyug School Admission 2024: Apply now | Admissions Open for 2024-25

Mar 26, 2024

NSSNET Result 2024, Check Navyug School Admission Test Here

Mar 22, 2024

NSSNET Admit Card 2024 (Out), Navyug School Entrance NTA Hall ticket

Mar 22, 2024

NSSNET Syllabus 2024, Download Navyug School Admission Test Syllabus

Mar 22, 2024

Diploma in Stenography - Duration, Eligibility, Top Colleges, Fees

Apr 18, 2024

Best Lab Technician Courses After 12th: Eligibility, Admission 2024, Colleges, Duration, Fees, Scope

Apr 16, 2024

What are Analytical Skills

Mar 21, 2024

Explore Premium

Mental Health

Understand Your Attachment Style And Learn How You Can Reform Your Relationships

7 min ⋆ Apr 09, 2023

Mental Health

7 Tips To Convey Your Struggles To Your Loved Ones

5 min ⋆ Mar 18, 2023

Mental Health

Decision-Making: Common Challenges Faced, Tips To Make Good Decisions

6 min ⋆ Feb 24, 2023

Parenting

How Stay-At-Home Parents Can Care For Themselves

6 min ⋆ Apr 20, 2023

Parenting

Teenage Relationships: Tips To Help Your Teenager Deal With A Breakup

6 min ⋆ Jan 13, 2023

Parenting

Getting Over The Pink and Blue Divide: Revising Gender Roles

4 min ⋆ Mar 05, 2022

Upcoming School Exams

National Institute of Open Schooling 12th Examination

Admit Card Date:28 March,2024 - 22 May,2024

Application Process

Exam Pattern

Admit Card

National Institute of Open Schooling 10th examination

Admit Card Date:28 March,2024 - 22 May,2024

Application Process

Exam Pattern

Mock Test

Punjab Board of Secondary Education 12th Examination

Exam Date:05 April,2024 - 27 April,2024

Exam Pattern

Result

Mock Test

National Means Cum-Merit Scholarship

Answer Key Date:16 April,2024 - 22 April,2024

Application Process

Exam Pattern

Mock Test

Goa Board Secondary School Certificate Examination

Exam Date:20 April,2024 - 20 April,2024

Exam Pattern

Mock Test

Admit Card

View All School Exams

Get answers from students and experts

Explore Career Options (By Industry)

Data Administrator

Database professionals use software to store and organise data such as financial information, and customer shipping records. Individuals who opt for a career as data administrators ensure that data is available for users and secured from unauthorised sales. DB administrators may work in various types of industries. It may involve computer systems design, service firms, insurance companies, banks and hospitals.

4 Jobs Available

Bio Medical Engineer

The field of biomedical engineering opens up a universe of expert chances. An Individual in the biomedical engineering career path work in the field of engineering as well as medicine, in order to find out solutions to common problems of the two fields. The biomedical engineering job opportunities are to collaborate with doctors and researchers to develop medical systems, equipment, or devices that can solve clinical problems. Here we will be discussing jobs after biomedical engineering, how to get a job in biomedical engineering, biomedical engineering scope, and salary.

4 Jobs Available

Ethical Hacker

A career as ethical hacker involves various challenges and provides lucrative opportunities in the digital era where every giant business and startup owns its cyberspace on the world wide web. Individuals in the ethical hacker career path try to find the vulnerabilities in the cyber system to get its authority. If he or she succeeds in it then he or she gets its illegal authority. Individuals in the ethical hacker career path then steal information or delete the file that could affect the business, functioning, or services of the organization.

3 Jobs Available

GIS Expert

GIS officer work on various GIS software to conduct a study and gather spatial and non-spatial information. GIS experts update the GIS data and maintain it. The databases include aerial or satellite imagery, latitudinal and longitudinal coordinates, and manually digitized images of maps. In a career as GIS expert, one is responsible for creating online and mobile maps.

3 Jobs Available

Data Analyst

The invention of the database has given fresh breath to the people involved in the data analytics career path. Analysis refers to splitting up a whole into its individual components for individual analysis. Data analysis is a method through which raw data are processed and transformed into information that would be beneficial for user strategic thinking.

Data are collected and examined to respond to questions, evaluate hypotheses or contradict theories. It is a tool for analyzing, transforming, modeling, and arranging data with useful knowledge, to assist in decision-making and methods, encompassing various strategies, and is used in different fields of business, research, and social science.

3 Jobs Available

Geothermal Engineer

Individuals who opt for a career as geothermal engineers are the professionals involved in the processing of geothermal energy. The responsibilities of geothermal engineers may vary depending on the workplace location. Those who work in fields design facilities to process and distribute geothermal energy. They oversee the functioning of machinery used in the field.

3 Jobs Available

Database Architect

If you are intrigued by the programming world and are interested in developing communications networks then a career as database architect may be a good option for you. Data architect roles and responsibilities include building design models for data communication networks. Wide Area Networks (WANs), local area networks (LANs), and intranets are included in the database networks. It is expected that database architects will have in-depth knowledge of a company's business to develop a network to fulfil the requirements of the organisation. Stay tuned as we look at the larger picture and give you more information on what is db architecture, why you should pursue database architecture, what to expect from such a degree and what your job opportunities will be after graduation. Here, we will be discussing how to become a data architect. Students can visit NIT Trichy, IIT Kharagpur, JMI New Delhi.

3 Jobs Available

Remote Sensing Technician

Individuals who opt for a career as a remote sensing technician possess unique personalities. Remote sensing analysts seem to be rational human beings, they are strong, independent, persistent, sincere, realistic and resourceful. Some of them are analytical as well, which means they are intelligent, introspective and inquisitive.

Remote sensing scientists use remote sensing technology to support scientists in fields such as community planning, flight planning or the management of natural resources. Analysing data collected from aircraft, satellites or ground-based platforms using statistical analysis software, image analysis software or Geographic Information Systems (GIS) is a significant part of their work. Do you want to learn how to become remote sensing technician? There's no need to be concerned; we've devised a simple remote sensing technician career path for you. Scroll through the pages and read.

3 Jobs Available

Budget Analyst

Budget analysis, in a nutshell, entails thoroughly analyzing the details of a financial budget. The budget analysis aims to better understand and manage revenue. Budget analysts assist in the achievement of financial targets, the preservation of profitability, and the pursuit of long-term growth for a business. Budget analysts generally have a bachelor's degree in accounting, finance, economics, or a closely related field. Knowledge of Financial Management is of prime importance in this career.

4 Jobs Available

Data Analyst

3 Jobs Available

Underwriter

An underwriter is a person who assesses and evaluates the risk of insurance in his or her field like mortgage, loan, health policy, investment, and so on and so forth. The underwriter career path does involve risks as analysing the risks means finding out if there is a way for the insurance underwriter jobs to recover the money from its clients. If the risk turns out to be too much for the company then in the future it is an underwriter who will be held accountable for it. Therefore, one must carry out his or her job with a lot of attention and diligence.

3 Jobs Available

Finance Executive

3 Jobs Available

Product Manager

A Product Manager is a professional responsible for product planning and marketing. He or she manages the product throughout the Product Life Cycle, gathering and prioritising the product. A product manager job description includes defining the product vision and working closely with team members of other departments to deliver winning products.

3 Jobs Available

Operations Manager

Individuals in the operations manager jobs are responsible for ensuring the efficiency of each department to acquire its optimal goal. They plan the use of resources and distribution of materials. The operations manager's job description includes managing budgets, negotiating contracts, and performing administrative tasks.

3 Jobs Available

Stock Analyst

Individuals who opt for a career as a stock analyst examine the company's investments makes decisions and keep track of financial securities. The nature of such investments will differ from one business to the next. Individuals in the stock analyst career use data mining to forecast a company's profits and revenues, advise clients on whether to buy or sell, participate in seminars, and discussing financial matters with executives and evaluate annual reports.

2 Jobs Available

Researcher

A Researcher is a professional who is responsible for collecting data and information by reviewing the literature and conducting experiments and surveys. He or she uses various methodological processes to provide accurate data and information that is utilised by academicians and other industry professionals. Here, we will discuss what is a researcher, the researcher's salary, types of researchers.

2 Jobs Available

Welding Engineer

Welding Engineer Job Description: A Welding Engineer work involves managing welding projects and supervising welding teams. He or she is responsible for reviewing welding procedures, processes and documentation. A career as Welding Engineer involves conducting failure analyses and causes on welding issues.

5 Jobs Available

Transportation Planner

A career as Transportation Planner requires technical application of science and technology in engineering, particularly the concepts, equipment and technologies involved in the production of products and services. In fields like land use, infrastructure review, ecological standards and street design, he or she considers issues of health, environment and performance. A Transportation Planner assigns resources for implementing and designing programmes. He or she is responsible for assessing needs, preparing plans and forecasts and compliance with regulations.

3 Jobs Available

Environmental Engineer

Individuals who opt for a career as an environmental engineer are construction professionals who utilise the skills and knowledge of biology, soil science, chemistry and the concept of engineering to design and develop projects that serve as solutions to various environmental problems.

2 Jobs Available

Safety Manager

A Safety Manager is a professional responsible for employee’s safety at work. He or she plans, implements and oversees the company’s employee safety. A Safety Manager ensures compliance and adherence to Occupational Health and Safety (OHS) guidelines.

2 Jobs Available

Conservation Architect

A Conservation Architect is a professional responsible for conserving and restoring buildings or monuments having a historic value. He or she applies techniques to document and stabilise the object’s state without any further damage. A Conservation Architect restores the monuments and heritage buildings to bring them back to their original state.

2 Jobs Available

Structural Engineer

A Structural Engineer designs buildings, bridges, and other related structures. He or she analyzes the structures and makes sure the structures are strong enough to be used by the people. A career as a Structural Engineer requires working in the construction process. It comes under the civil engineering discipline. A Structure Engineer creates structural models with the help of computer-aided design software.

2 Jobs Available

Highway Engineer

Highway Engineer Job Description: A Highway Engineer is a civil engineer who specialises in planning and building thousands of miles of roads that support connectivity and allow transportation across the country. He or she ensures that traffic management schemes are effectively planned concerning economic sustainability and successful implementation.

2 Jobs Available

Field Surveyor

Are you searching for a Field Surveyor Job Description? A Field Surveyor is a professional responsible for conducting field surveys for various places or geographical conditions. He or she collects the required data and information as per the instructions given by senior officials.

2 Jobs Available

Orthotist and Prosthetist

Orthotists and Prosthetists are professionals who provide aid to patients with disabilities. They fix them to artificial limbs (prosthetics) and help them to regain stability. There are times when people lose their limbs in an accident. In some other occasions, they are born without a limb or orthopaedic impairment. Orthotists and prosthetists play a crucial role in their lives with fixing them to assistive devices and provide mobility.

6 Jobs Available

Pathologist

A career in pathology in India is filled with several responsibilities as it is a medical branch and affects human lives. The demand for pathologists has been increasing over the past few years as people are getting more aware of different diseases. Not only that, but an increase in population and lifestyle changes have also contributed to the increase in a pathologist’s demand. The pathology careers provide an extremely huge number of opportunities and if you want to be a part of the medical field you can consider being a pathologist. If you want to know more about a career in pathology in India then continue reading this article.

5 Jobs Available

Veterinary Doctor

5 Jobs Available

Speech Therapist

4 Jobs Available

Gynaecologist

Gynaecology can be defined as the study of the female body. The job outlook for gynaecology is excellent since there is evergreen demand for one because of their responsibility of dealing with not only women’s health but also fertility and pregnancy issues. Although most women prefer to have a women obstetrician gynaecologist as their doctor, men also explore a career as a gynaecologist and there are ample amounts of male doctors in the field who are gynaecologists and aid women during delivery and childbirth.

4 Jobs Available

Audiologist

The audiologist career involves audiology professionals who are responsible to treat hearing loss and proactively preventing the relevant damage. Individuals who opt for a career as an audiologist use various testing strategies with the aim to determine if someone has a normal sensitivity to sounds or not. After the identification of hearing loss, a hearing doctor is required to determine which sections of the hearing are affected, to what extent they are affected, and where the wound causing the hearing loss is found. As soon as the hearing loss is identified, the patients are provided with recommendations for interventions and rehabilitation such as hearing aids, cochlear implants, and appropriate medical referrals. While audiology is a branch of science that studies and researches hearing, balance, and related disorders.

3 Jobs Available

Oncologist

An oncologist is a specialised doctor responsible for providing medical care to patients diagnosed with cancer. He or she uses several therapies to control the cancer and its effect on the human body such as chemotherapy, immunotherapy, radiation therapy and biopsy. An oncologist designs a treatment plan based on a pathology report after diagnosing the type of cancer and where it is spreading inside the body.

3 Jobs Available

Anatomist

Are you searching for an ‘Anatomist job description’? An Anatomist is a research professional who applies the laws of biological science to determine the ability of bodies of various living organisms including animals and humans to regenerate the damaged or destroyed organs. If you want to know what does an anatomist do, then read the entire article, where we will answer all your questions.

2 Jobs Available

Actor

For an individual who opts for a career as an actor, the primary responsibility is to completely speak to the character he or she is playing and to persuade the crowd that the character is genuine by connecting with them and bringing them into the story. This applies to significant roles and littler parts, as all roles join to make an effective creation. Here in this article, we will discuss how to become an actor in India, actor exams, actor salary in India, and actor jobs.

4 Jobs Available

Acrobat

Individuals who opt for a career as acrobats create and direct original routines for themselves, in addition to developing interpretations of existing routines. The work of circus acrobats can be seen in a variety of performance settings, including circus, reality shows, sports events like the Olympics, movies and commercials. Individuals who opt for a career as acrobats must be prepared to face rejections and intermittent periods of work. The creativity of acrobats may extend to other aspects of the performance. For example, acrobats in the circus may work with gym trainers, celebrities or collaborate with other professionals to enhance such performance elements as costume and or maybe at the teaching end of the career.

3 Jobs Available

Video Game Designer

Career as a video game designer is filled with excitement as well as responsibilities. A video game designer is someone who is involved in the process of creating a game from day one. He or she is responsible for fulfilling duties like designing the character of the game, the several levels involved, plot, art and similar other elements. Individuals who opt for a career as a video game designer may also write the codes for the game using different programming languages.

Depending on the video game designer job description and experience they may also have to lead a team and do the early testing of the game in order to suggest changes and find loopholes.

3 Jobs Available

Radio Jockey

Radio Jockey is an exciting, promising career and a great challenge for music lovers. If you are really interested in a career as radio jockey, then it is very important for an RJ to have an automatic, fun, and friendly personality. If you want to get a job done in this field, a strong command of the language and a good voice are always good things. Apart from this, in order to be a good radio jockey, you will also listen to good radio jockeys so that you can understand their style and later make your own by practicing.

A career as radio jockey has a lot to offer to deserving candidates. If you want to know more about a career as radio jockey, and how to become a radio jockey then continue reading the article.

3 Jobs Available

Choreographer

The word “choreography" actually comes from Greek words that mean “dance writing." Individuals who opt for a career as a choreographer create and direct original dances, in addition to developing interpretations of existing dances. A Choreographer dances and utilises his or her creativity in other aspects of dance performance. For example, he or she may work with the music director to select music or collaborate with other famous choreographers to enhance such performance elements as lighting, costume and set design.

2 Jobs Available

Social Media Manager

A career as social media manager involves implementing the company’s or brand’s marketing plan across all social media channels. Social media managers help in building or improving a brand’s or a company’s website traffic, build brand awareness, create and implement marketing and brand strategy. Social media managers are key to important social communication as well.

2 Jobs Available

Photographer

Photography is considered both a science and an art, an artistic means of expression in which the camera replaces the pen. In a career as a photographer, an individual is hired to capture the moments of public and private events, such as press conferences or weddings, or may also work inside a studio, where people go to get their picture clicked. Photography is divided into many streams each generating numerous career opportunities in photography. With the boom in advertising, media, and the fashion industry, photography has emerged as a lucrative and thrilling career option for many Indian youths.

2 Jobs Available

Producer

An individual who is pursuing a career as a producer is responsible for managing the business aspects of production. They are involved in each aspect of production from its inception to deception. Famous movie producers review the script, recommend changes and visualise the story.

They are responsible for overseeing the finance involved in the project and distributing the film for broadcasting on various platforms. A career as a producer is quite fulfilling as well as exhaustive in terms of playing different roles in order for a production to be successful. Famous movie producers are responsible for hiring creative and technical personnel on contract basis.

2 Jobs Available

Copy Writer

In a career as a copywriter, one has to consult with the client and understand the brief well. A career as a copywriter has a lot to offer to deserving candidates. Several new mediums of advertising are opening therefore making it a lucrative career choice. Students can pursue various copywriter courses such as Journalism, Advertising, Marketing Management. Here, we have discussed how to become a freelance copywriter, copywriter career path, how to become a copywriter in India, and copywriting career outlook.

5 Jobs Available

Vlogger

In a career as a vlogger, one generally works for himself or herself. However, once an individual has gained viewership there are several brands and companies that approach them for paid collaboration. It is one of those fields where an individual can earn well while following his or her passion.

Ever since internet costs got reduced the viewership for these types of content has increased on a large scale. Therefore, a career as a vlogger has a lot to offer. If you want to know more about the Vlogger eligibility, roles and responsibilities then continue reading the article.

3 Jobs Available

Publisher

For publishing books, newspapers, magazines and digital material, editorial and commercial strategies are set by publishers. Individuals in publishing career paths make choices about the markets their businesses will reach and the type of content that their audience will be served. Individuals in book publisher careers collaborate with editorial staff, designers, authors, and freelance contributors who develop and manage the creation of content.

3 Jobs Available

Journalist

Careers in journalism are filled with excitement as well as responsibilities. One cannot afford to miss out on the details. As it is the small details that provide insights into a story. Depending on those insights a journalist goes about writing a news article. A journalism career can be stressful at times but if you are someone who is passionate about it then it is the right choice for you. If you want to know more about the media field and journalist career then continue reading this article.

3 Jobs Available

Editor

Individuals in the editor career path is an unsung hero of the news industry who polishes the language of the news stories provided by stringers, reporters, copywriters and content writers and also news agencies. Individuals who opt for a career as an editor make it more persuasive, concise and clear for readers. In this article, we will discuss the details of the editor's career path such as how to become an editor in India, editor salary in India and editor skills and qualities.

3 Jobs Available

Reporter

Individuals who opt for a career as a reporter may often be at work on national holidays and festivities. He or she pitches various story ideas and covers news stories in risky situations. Students can pursue a BMC (Bachelor of Mass Communication), B.M.M. (Bachelor of Mass Media), or MAJMC (MA in Journalism and Mass Communication) to become a reporter. While we sit at home reporters travel to locations to collect information that carries a news value.

2 Jobs Available

Corporate Executive

Are you searching for a Corporate Executive job description? A Corporate Executive role comes with administrative duties. He or she provides support to the leadership of the organisation. A Corporate Executive fulfils the business purpose and ensures its financial stability. In this article, we are going to discuss how to become corporate executive.

2 Jobs Available

Multimedia Specialist

A multimedia specialist is a media professional who creates, audio, videos, graphic image files, computer animations for multimedia applications. He or she is responsible for planning, producing, and maintaining websites and applications.

2 Jobs Available

Welding Engineer

5 Jobs Available

QA Manager

4 Jobs Available

Quality Controller

A quality controller plays a crucial role in an organisation. He or she is responsible for performing quality checks on manufactured products. He or she identifies the defects in a product and rejects the product.

A quality controller records detailed information about products with defects and sends it to the supervisor or plant manager to take necessary actions to improve the production process.

3 Jobs Available

Production Manager

3 Jobs Available

Product Manager

3 Jobs Available

QA Lead

A QA Lead is in charge of the QA Team. The role of QA Lead comes with the responsibility of assessing services and products in order to determine that he or she meets the quality standards. He or she develops, implements and manages test plans.

2 Jobs Available

Structural Engineer

2 Jobs Available

Process Development Engineer

The Process Development Engineers design, implement, manufacture, mine, and other production systems using technical knowledge and expertise in the industry. They use computer modeling software to test technologies and machinery. An individual who is opting career as Process Development Engineer is responsible for developing cost-effective and efficient processes. They also monitor the production process and ensure it functions smoothly and efficiently.

2 Jobs Available

QA Manager

4 Jobs Available

AWS Solution Architect

An AWS Solution Architect is someone who specializes in developing and implementing cloud computing systems. He or she has a good understanding of the various aspects of cloud computing and can confidently deploy and manage their systems. He or she troubleshoots the issues and evaluates the risk from the third party.

4 Jobs Available

Azure Administrator

An Azure Administrator is a professional responsible for implementing, monitoring, and maintaining Azure Solutions. He or she manages cloud infrastructure service instances and various cloud servers as well as sets up public and private cloud systems.

4 Jobs Available

Computer Programmer

Careers in computer programming primarily refer to the systematic act of writing code and moreover include wider computer science areas. The word 'programmer' or 'coder' has entered into practice with the growing number of newly self-taught tech enthusiasts. Computer programming careers involve the use of designs created by software developers and engineers and transforming them into commands that can be implemented by computers. These commands result in regular usage of social media sites, word-processing applications and browsers.

3 Jobs Available

Product Manager

3 Jobs Available

Information Security Manager

Individuals in the information security manager career path involves in overseeing and controlling all aspects of computer security. The IT security manager job description includes planning and carrying out security measures to protect the business data and information from corruption, theft, unauthorised access, and deliberate attack

3 Jobs Available

ITSM Manager

3 Jobs Available

Automation Test Engineer

An Automation Test Engineer job involves executing automated test scripts. He or she identifies the project’s problems and troubleshoots them. The role involves documenting the defect using management tools. He or she works with the application team in order to resolve any issues arising during the testing process.

2 Jobs Available

Everything about Education

Latest updates, Exclusive Content, Webinars and more.

Subscribe to Premium

Download Careers360 App's

Regular exam updates, QnA, Predictors, College Applications & E-books now on your Mobile

250M+
Students
30,000+
Colleges
500+
Exams
1500+
E-Books
12000+
Cetifications

We Appeared in

Explore Top Colleges Accepting Applications

JEE Test Series

Applications Open

Option 1) $0.34\; J$	Option 2) $0.16\; J$
Option 3) $1.00\; J$	Option 4) $0.67\; J$

Option 1) $2,000 \; J - 5,000\; J$	Option 2) $200 \, \, J - 500 \, \, J$
Option 3) $2\times 10^{5}J-3\times 10^{5}J$	Option 4) $20,000 \, \, J - 50,000 \, \, J$

Option 1) $K/2\,$	Option 2) $\; K\;$
Option 3) $zero\;$	Option 4) $K/4$

Option 1) $11.2\, L\, H_{2(g)}$ at STP is produced for every mole $HCL_{(aq)}$ consumed	Option 2) $6L\, HCl_{(aq)}$ is consumed for ever $3L\, H_{2(g)}$ produced
Option 3) $33.6 L\, H_{2(g)}$ is produced regardless of temperature and pressure for every mole Al that reacts	Option 4) $67.2\, L\, H_{2(g)}$ at STP is produced for every mole Al that reacts .

Option 1) 0.02	Option 2) 3.125 × 10^-2
Option 3) 1.25 × 10^-2	Option 4) 2.5 × 10^-2

Option 1) decrease twice	Option 2) increase two fold
Option 3) remain unchanged	Option 4) be a function of the molecular mass of the substance.

Option 1) Molality	Option 2) Weight fraction of solute
Option 3) Fraction of solute present in water	Option 4) Mole fraction.

Option 1) twice that in 60 g carbon	Option 2) 6.023 × 10²²
Option 3) half that in 8 g He	Option 4) 558.5 × 6.023 × 10²³

Option 1) less than 3	Option 2) more than 3 but less than 6
Option 3) more than 6 but less than 9	Option 4) more than 9

Exams

Ranking

Products & Resources

NCERT Solutions

Exams

Colleges

Predictors

Resources

Exams

Colleges & Courses

Predictors

Resources

Exams

Colleges

Predictors

Resources

Exams

Colleges

Predictors & E-Books

Resources

Exams

Animation Courses

Colleges

Resources

Exams

Colleges

Resources

Exams

Resources

Top Courses & Careers

Colleges

Exams

Colleges

Resources

Quick Links

Exams

Colleges

Resources

Exams

Colleges

Resources

Diploma Colleges

Exams

Resources

Upcoming Events

Other Exams

Exams

Colleges

Top Countries

Student Visas

Exams

Colleges

Upcoming Events

Resources

Engineering Preparation

Medical Preparation

Online Courses

Products

Top Streams

Specializations

Resources

Top Providers

NCERT Solutions for Class 11 Chemistry Chapter 5 States of Matter

NCERT Solutions for Class 11 Chemistry Chapter 5 States of Matter- Exercise Questions

Frequently Asked Question (FAQs)

Also Read

Articles

Explore Premium

Understand Your Attachment Style And Learn How You Can Reform Your Relationships

7 Tips To Convey Your Struggles To Your Loved Ones

Decision-Making: Common Challenges Faced, Tips To Make Good Decisions

How Stay-At-Home Parents Can Care For Themselves

Teenage Relationships: Tips To Help Your Teenager Deal With A Breakup

Getting Over The Pink and Blue Divide: Revising Gender Roles

Upcoming School Exams

National Institute of Open Schooling 12th Examination

National Institute of Open Schooling 10th examination

Punjab Board of Secondary Education 12th Examination

National Means Cum-Merit Scholarship

Goa Board Secondary School Certificate Examination