Browse by Stream
QnA
Home
QnA
Go To Your Study Dashboard

Get Answers to all your Questions

header-bg qa
  • Home
  • NCERT
  • Using mean value theorem, prove that there is point on the curve y = 2x2 - 5x + 3 between the points A(1, 0) and B(2, 1), where tangent is parallel to the chord A. Also, find that point.

Using the mean value theorem, prove that there is a point on the curve 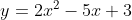y = 2x^2 - 5x + 3 between the points A(1,0) and B(2, 1), where the tangent is parallel to the chord AB. Also, find that point.

 

Answers (1)

Solution

Given:  y = 2x^2 - 5x + 3 in [1,2]

Now, we have to show that f(x) verify the Mean Value Theorem

First of all, Conditions of Mean Value theorem are:

a) f(x) is continuous at (a,b)

b) f(x) is derivable at (a,b)

If both the conditions are satisfied, then there exist some ‘c’ in (a,b) such that

f^{\prime}(c)=\frac{f(b)-f(a)}{b-a}

Condition 1:

y=2 x^{2}-5 x+3
since,  f(x)  is a polynomial and we know that, every polynomial function is continuous for all x \in R
\Rightarrow y=2 x^{2}-5 x+3 is continuous at x \in[1,2]
Hence, condition 1 is satisfied.
Condition 2
y=2 x^{2}-5 x+3
since,  f(x) is a polynomial and every polynomial function is differentiable for all x \in R
y^{\prime}=4 x-5
\Rightarrow \mathrm{y}=2 \mathrm{x}^{3}-5 \mathrm{x}+3 is differentiable at [1, 2]
Hence, condition 2 is satisfied.
Thus, Mean Value Theorem is applicable to the given function.
Now,
\\f(x)=y=2 x^{2}-5 x+3 x \in[1,2] \\ f(a)=f(1)=2(1)^{2}-5(1)+3=2-5+3=0

f(b)=f(2)=2(2)^{2}-5(2)+3=8-10+3=1
Then, there exist c \in(0,1) such that
\mathrm{f}^{\prime}(\mathrm{c})=\frac{\mathrm{f}(\mathrm{b})-\mathrm{f}(\mathrm{a})}{\mathrm{b}-\mathrm{a}}
Put  x=c  in equation, we get
y^{\prime}=4 c-5 \ldots(i)

By Mean Value Theorem,
\\\mathrm{f}^{\prime}(\mathrm{c})=\frac{\mathrm{f}(\mathrm{b})-\mathrm{f}(\mathrm{a})}{\mathrm{b}-\mathrm{a}} \\4 c-5=\frac{f(2)-f(1)}{2-1} \\\Rightarrow 4 c-5=\frac{1-0}{1} \\\Rightarrow 4 c-5=1 \\\Rightarrow 4 c=6 \\\Rightarrow c=\frac{6}{4}=\frac{3}{2}
So, value of  c=\frac{3}{2} \in(1,2)
\Rightarrow x=\frac{3}{2}
Thus, Mean Value Theorem is verified.
Put x=\frac{3}{2} in given equation y=2 x^{2}-5 x+3, we have

\begin{aligned} &y=2\left(\frac{3}{2}\right)^{2}-5\left(\frac{3}{2}\right)+3\\ &\Rightarrow y=\frac{9}{2}-\frac{15}{2}+3\\ &\Rightarrow y=\frac{9-15+6}{2}\\ &\Rightarrow y=0\\ &\text { Hence, the tangent to the curve is parallel to the chord AB at }\\ &\left(\frac{3}{2}, 0\right) \end{aligned}

 

Posted by

infoexpert22

View full answer

Similar Questions

Latest Question