Browse by Stream
QnA
Home
QnA
Go To Your Study Dashboard

Get Answers to all your Questions

header-bg qa
  • Home
  • Engineering
  • There are two infinitely long straight current carrying conductors and they are held at right angles to each other so that their common ends meet at the origin as shown in the figure given below. The ratio of current in both conductors is <img alt="1: 1"

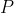There are two infinitely long straight current carrying conductors and they are held at right angles to each other so that their common ends meet at the origin as shown in the figure given below. The ratio of current in both conductors is 1: 1. The magnetic field at point P is______.    
Option: 1 \begin{aligned} &\frac{\mu_{0} \mathrm{I}}{4 \pi x y}\left[\sqrt{x^{2}+y^{2}}-(x+y)\right] \\ \end{aligned}
Option: 2 \frac{\mu_{0} \mathrm{I} x y}{4 \pi}\left[\sqrt{x^{2}+y^{2}}-(x+y)\right] \\
Option: 3 \frac{\mu_{0} \mathrm{I}}{4 \pi x y}\left[\sqrt{x^{2}+y^{2}}+(x+y)\right] \\
Option: 4 \frac{\mu_{0} \mathrm{I} x y}{4 \pi}\left[\sqrt{x^{2}+y^{2}}+(x+y)\right]

Answers (1)

best_answer

B_{1}= \frac{\mu _{0}I}{4\pi x}\left [ \sin \theta _{2} +\sin 90^{\circ}\right ]\otimes

B_{1}= \frac{\mu _{0}I}{4\pi x}\left [ \sin \theta _{2} +1\right ]\otimes
B_{2}= \frac{\mu _{0}I}{4\pi y}\left [ \sin \theta _{1} +\sin 90^{\circ}\right ]\otimes
B_{2}= \frac{\mu _{0}I}{4\pi y}\left [ 1+\sin \theta _{1}\right ]\otimes

B_{total}=B_{1}+B_{2}
=\frac{\mu _{0}I}{4\pi }\left [ \frac{\left ( 1+\sin \theta _{2} \right )}{x}+\frac{\left ( 1+\sin \theta _{1} \right )}{y} \right ]


\sin \theta _{2}= \frac{y}{\sqrt{x^{2}+y^{2}}}\: \sin \theta _{1}= \frac{x}{\sqrt{x^{2}+y^{2}}}
= \frac{\mu _{0}I}{4\pi }\left [ \frac{1}{x}+\frac{y}{x\sqrt{x^{2}+y^{2}}}+\frac{1}{y}+\frac{x}{y\sqrt{x^{2}+y^{2}}} \right ]
= \frac{\mu _{0}I}{4\pi }\left [ \frac{y\sqrt{x^{2}+y^{2}}+y^{2}+x\sqrt{x^{2}+y^{2}}+x^{2}}{xy\sqrt{x^{2}+y^{2}}} \right ]
B_{total}= \frac{\mu _{0}I}{4\pi xy }\left [ \left ( x+y \right )+\sqrt{x^{2}+y^{2}} \right ]\otimes
The correct option is (3)


 

Posted by

vishal kumar

View full answer

JEE Main high-scoring chapters and topics

Study 40% syllabus and score up to 100% marks in JEE

Similar Questions

Trending Articles/News

anam-khan

JEE Main 2025 response sheet errors: Delhi HC allows student to apply for JEE Adv, seeks NTA explanation
anam-khan

Four Kashmiri girls defy the odds, score 99+ percentile in JEE Main 2025
anam-khan

JEE, NEET, CUET coaching centres: Assam, Rajasthan have framed laws, but will they work?

Latest Question