-
Engineering and Architecture
Exams
Colleges
Predictors
Resources
-
Computer Application and IT
Quick Links
Colleges
-
Pharmacy
Colleges
Resources
-
Hospitality and Tourism
Colleges
Resources
Diploma Colleges
-
Competition
Other Exams
Resources
-
School
Exams
Top Schools
Products & Resources
-
Study Abroad
Top Countries
Resources
-
Arts, Commerce & Sciences
Exams
Colleges
Upcoming Events
Resources
-
Management and Business Administration
Colleges & Courses
Predictors
-
Learn
Law Preparation
MBA Preparation
Engineering Preparation
Medical Preparation
-
Online Courses and Certifications
Top Streams
Specializations
- Digital Marketing Certification Courses
- Cyber Security Certification Courses
- Artificial Intelligence Certification Courses
- Business Analytics Certification Courses
- Data Science Certification Courses
- Cloud Computing Certification Courses
- Machine Learning Certification Courses
- View All Certification Courses
Resources
-
Medicine and Allied Sciences
Colleges
Predictors
Resources
-
Law
Resources
Colleges
-
Animation and Design
Exams
Predictors & Articles
Colleges
Resources
-
Media, Mass Communication and Journalism
Colleges
Resources
-
Finance & Accounts
Top Courses & Careers
Colleges
Get Answers to all your Questions


All Questions
Let the volume of a parallelopiped whose coterminous edges are given by and
be 1 cu. unit. If
be the angle between the edges
and
then
can be :
Option: 1
Option: 2
Option: 3
Option: 4
Dot (Scalar) Product in Terms of Components -
Angle between two vectors
-
Geometrical Interpretation of Scalar Triple Product -
Let vectors and
represented the sides of a parallelepiped OA, OB and OC respectively. Then,
is a vector perpendicular to the plane of
and
. Let ? be the angle between vectors
and
and α be the angle between
and
.
If is a unit vector along
, then α is the angle between
and
.

-
Correct Option (3)
View Full Answer(1)Let and
be two vectors. If
is a vector such that
and
then
is equal to :
Option: 1
Option: 2
Option: 3
Option: 4
Vector Triple Product -
For three vectors and
vector triple product is defined as
.
-
Correct Option (3)
View Full Answer(1)Crack CUET with india's "Best Teachers"
- HD Video Lectures
- Unlimited Mock Tests
- Faculty Support

Let and
be three unit vectors such that
.If
and
then the ordered pair,
is equal to :
Option: 1
Option:2
Option: 3
Option: 4
Addition and subtraction of Vectors -
Properties of vector addition
The sum of two vectors is always a vector.
Properties of vector Subtraction
-
Correct Option (3)
View Full Answer(1)Let be three mutually perpendicular vectors of the same magnitude and equally inclined at an angle
, with the vector
. Then
is equal to ________.
Given,
and these are mutually perpendicular,
So,
Angle of with
Now,
Using this in (i)
Hence, the correct answer is 4
View Full Answer(1)JEE Main high-scoring chapters and topics
Study 40% syllabus and score up to 100% marks in JEE
Let . Let a vector
be in the plane containing
. If
is perpendicular to the vector
and its projection on
is 19 units, then
is equal to___________
(a vector in the plane of &
and perpendicular to
)
Let 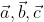 be three vectors mutually perpendicular to each other and have same magnitude. If a vector
be three vectors mutually perpendicular to each other and have same magnitude. If a vector 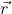 satisfies
satisfies
 then
then 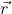 is equal to :
is equal to :
Option: 1 
Option: 2 
Option: 3 
Option: 4 
Give expression is
NEET 2024 Most scoring concepts
- Just Study 32% of the NEET syllabus and Score up to 100% marks
Let 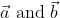 be two vectors such that
be two vectors such that  and the angle between
and the angle between 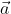 and
and 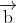 is
is 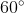 . If
. If 
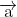 is a unit vector, then
is a unit vector, then 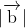 is equal to :
is equal to :
Option: 1 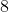
Option: 2 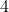
Option: 3 
Option: 4 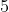
OR
option (4)
The angle between the straight lines, whose direction cosines are given by the equations  , is :
, is :
Option: 1 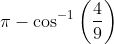
Option: 2 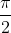
Option: 3 
Option: 4 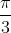
...............(1)
..........(2)
From (1) and (2)
So
Also
So
Angle
Crack CUET with india's "Best Teachers"
- HD Video Lectures
- Unlimited Mock Tests
- Faculty Support

Let be three vectors such that,
is perpendicular to
. Then the greatest amongst the values of
is__________
If the projection of the vector on the sum of the two vectors
and
is 1, then
is equal to ________.
Sum of vectors ( ) is
Projection of on
is
JEE Main high-scoring chapters and topics
Study 40% syllabus and score up to 100% marks in JEE


