Get Answers to all your Questions

Filter By

Clear Filter

All Questions

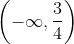Let 'a' be a real number such that the function $\mathrm{f}(\mathrm{x})=\mathrm{ax}^{2}+6 \mathrm{x}-15, \mathrm{x} \in \mathbf{R}$ is increasing in $\left(-\infty, \frac{3}{4}\right)$ and decreasing in 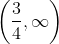$\left(\frac{3}{4}, \infty\right)$ . Then the function $g(x)=a x^{2}-6 x+15, x \in \mathbf{R}$ has a

Option: 1 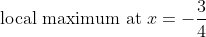$\text {local maximum at}\; x=-\frac{3}{4}$
Option: 2 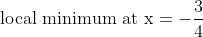$\text {local minimum at }\mathrm{x}=-\frac{3}{4}$
Option: 3 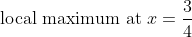$\text {local maximum at} \; x=\frac{3}{4}$
Option: 4 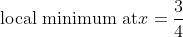$\text {local minimum at} x=\frac{3}{4}$

$\begin{aligned} &f(x)=a x^{2}+6 x-15 . \\ &f^{\prime}(x)=2 a x+6 . \end{aligned}$

$f(x)$ increasing in $\left(-\infty, \frac{3}{4}\right)$ & decreasing in $\left(\frac{3}{4}, \infty\right)$

$f^{\prime}(x)>0 \text { in }\left(-\infty, \frac{3}{4}\right) \& f^{\prime}(x)<0 \text { in }\left(\frac{3}{4}, \infty\right)$

As $f'(x)$ is linear, so $f^{\prime}(x)=0 \text { at } x=\frac{3}{4}$

$\begin{aligned} &\Rightarrow \quad 2 \cdot a \cdot\left(\frac{3}{4}\right)+6=0 \\ &\Rightarrow \quad a=-4 . \end{aligned}$

Now, $g(x)=-4 x^{2}-6 x+15$

It is a downward parabola, so it will have maximum at $x=\frac{-b}{2 a} \text { (vertex) }$

So maximum at $x=\frac{-(-6)}{2 \cdot(-4)}=-\frac{3}{4}$

Hence, the correct answer is option (1)

View Full Answer(1)

Posted by

Kuldeep Maurya

Find the point on the curve $y^{2} = 4x$ which is nearest to the point (2, 1).

$$ Let $ (x,y) $ on the $ y^{2} = 4x $ is nearest point to the (2,1)$

$\\ $ Distance D = $ \sqrt{(x-2)^2+ (y-1)^2} \\ D = \sqrt{(\frac{y^2}{4}{}-2)^2+ (y-1)^2} \\ D = \sqrt{(y^2-8)^2+ 16(y-1)^2}/4 \\ \frac{dD}{dy} = 0 \\\\ \frac{2(y^2-8)(2y)+32(y-1)}{8\sqrt{(y^2-8)^2+ 16(y-1)^2}/4} = 0 \\\\ y^3-8y + 8y-8 = 0 \\\\ y = 2 \Rightarrow x =1 \\$

$\\\frac{d^2D}{dy^2}_{y = 2}>0$

y=2 has minima

$\\ $ Shortest Distance D = $ \sqrt{(1-2)^2+ (2-1)^2} = \sqrt{2} $ unit \\$

View Full Answer(1)

Posted by

Safeer PP

Crack CUET with india's "Best Teachers"

HD Video Lectures
Unlimited Mock Tests
Faculty Support

Find the slope of tangent to the curve $y = 2 cos^{2} (3x)$ at $x = \frac{\pi }{6}$

$y = 2 cos^{2} (3x)$

$\\\frac{dy}{dx} =- 2 \times 2 \times \cos (3x) \times \sin (3x) \times 3\\\\ \frac{dy}{dx}_{at x = \frac{\pi}{6}} =- 2 \times 2 \times \cos (\frac{\pi}{2}) \times \sin (\frac{\pi}{2}) \times 3 = 0$

Hence the slope of the tangent is zero.

View Full Answer(1)

Posted by

Safeer PP

Find the minimum value of (ax + by), where $xy = c^{2}$

$\\ \text { Given: } y=\frac{c^{2}}{x} \\ $Let P $ =a x+b y \\ P=a x+\frac{b c^{2}}{x} \\ $For critical point value $\frac{d P}{d x}= 0 \\ \frac{dP}{dx} = a-\frac{b c^{2}}{x^{2}}=0 \\ x^{2}=\frac{b c^{2}}{a} \Rightarrow x=\sqrt{\frac{b}{a}} \cdot c \\ $For minima $ \\ \frac{d^2P}{dx^2} = \frac{2bc^2}{x^3} \\ \\ \left.\frac{d^{2} P}{d x^{2}}\right|_{x=\sqrt{\frac{b}{a}}.c}=\frac{2 b c^{2}}{(\sqrt{\frac{b}{a}}.c)^{3}} \\=\frac{2 b }{c}\left[\sqrt{\frac{a}{b}} \right]^{3}>0$

$\\\text { At } x=\sqrt{\frac{b}{a}} \cdot c $ P has minimum value$

$\\$ minimum value of P = $ a \sqrt{\frac{b}{a}} \cdot c+ \frac{bc^{2}}{c} \sqrt{\frac{a}{b}}\\=2 \sqrt{a b} \cdot c$

View Full Answer(1)

Posted by

Safeer PP

JEE Main high-scoring chapters and topics

Study 40% syllabus and score up to 100% marks in JEE

Find the slope of the tangent to the curve $y = 2 \sin^{2} (3x) \text{at} x =\frac{\pi}{6} .$

$\\ y = 2 \sin^{2} (3x) \\$

$\\\frac{d y}{d x}=4 \sin 3 x \times \cos 3x \times 3 \\\\ \frac{dy }{dx}= 6 \sin 6x \\ \frac{dy }{dx}|_{\text{at} x = \frac{\pi}{6}}= 6 \sin \pi =0 \\\\ \text {Slope of tangent }=0$