Browse by Stream
QnA
Home
QnA
Go To Your Study Dashboard

Get Answers to all your Questions

header-bg qa
  • Home
  • Engineering
  • Let ABCD be a parallelogram such that <img alt="= \vec{q}" src="https

Let ABCD be a parallelogram such that \underset{AB}{\rightarrow} = \vec{q},    \underset{AD}{\rightarrow}  = \vec{p} and \angle BAD  be an acute angle. If   \vec{r} is the vector that coincides with the altitude directed from the vertex B to the side AD, then \vec{r} is given by

Option: 1

\vec{r}= 3\vec{q}-\frac{3\left ( \vec{p}.\vec{q} \right )}{\left ( \vec{p}.\vec{p} \right )}\vec{p}


Option: 2

\vec{r}= -\vec{q}+\left ( \frac{\vec{p}.\vec{q}}{\vec{p}.\vec{p}} \right )\vec{p}


Option: 3

\vec{r}= \vec{q}-\left ( \frac{\vec{p}.\vec{q}}{\vec{p}.\vec{p}} \right )\vec{p}


Option: 4

\vec{r}= -3\vec{q}+\frac{3\left ( \vec{p}.\vec{q} \right )}{\left ( \vec{p}.\vec{p} \right )}\vec{p}


Answers (1)

best_answer

\vec{r}=  \underset{BA}{\rightarrow} + \underset{AQ}{\rightarrow}

=-\vec{q}+projection\; of\; \underset{BA}{\rightarrow} across \underset{AD}{\rightarrow}

=-\vec{q}+\frac{(\vec{p}.\vec{q})\vec{p}}{(\vec{p}.\vec{p})}

As learnt in concept

Projection of vector b on vector a -

\vec{b}\cos \Theta = \frac{\vec{a}.\vec{b}}{\left | \vec{a} \right |}

- wherein

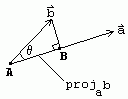
dot product

 

 

Posted by

SANGALDEEP SINGH

View full answer

JEE Main high-scoring chapters and topics

Study 40% syllabus and score up to 100% marks in JEE

Similar Questions