Browse by Stream
QnA
Home
QnA
Go To Your Study Dashboard

Get Answers to all your Questions

header-bg qa
  • Home
  • Engineering
  • On heating water, bubbles being formed at the bottom of the vessel detach and rise.Take the bubbles to be spheres of radius R and making a circular contact of radius r with the bottom of the vessel

On heating water, bubbles being formed at the bottom of the vessel detach and rise.Take the bubbles to be spheres of radius R and making a circular contact of radius r with the bottom of the vessel. If r << R, and the surface tension of water is T, value of r just before bubbles detach is :(density of water is \rho _w )

Option: 1

R^{2}\sqrt{\frac{2p_{w}g}{3T}}


Option: 2

R^{2}\sqrt{\frac{p_{w}g}{6T}}


Option: 3

R^{2}\sqrt{\frac{p_{w}g}{T}}


Option: 4

R^{2}\sqrt{\frac{3p_{w}g}{T}}


Answers (1)

best_answer

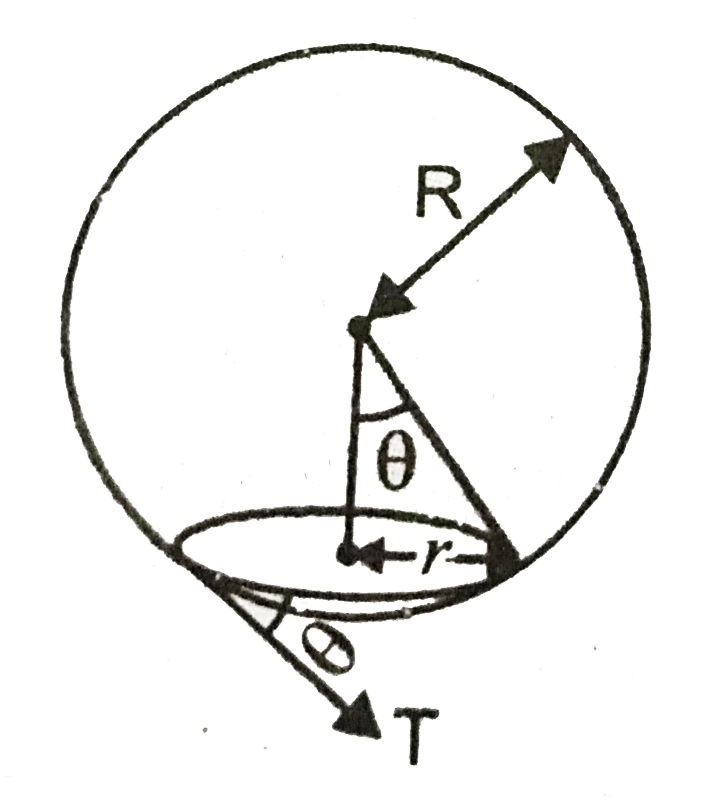
Here T is the surface tension and we know that surface tension,

 T=\frac{F}{l}=F=Tl=T(2\pi r),

where F is the force due to surface tension and its vertical component will be T(2\pi r)Sin\theta.

Now The bubble will detach, if Buoyant force ≥ vertical component of surface tension force, so for the bubble to just detach:-

vertical component of surface tension force=buoyant force(or upthrust)

T(2\pi r)Sin\theta=V\rho_wg\\\Rightarrow T(2\pi r)\frac{r}{R}=\frac{4}{3}\pi R^3\rho_wg\\ \Rightarrow r^2=\frac{2R^4\rho_wg}{3T}\\ \Rightarrow r=R^2\sqrt{\frac{2\rho_wg}{3T}}

Posted by

sudhir.kumar

View full answer

JEE Main high-scoring chapters and topics

Study 40% syllabus and score up to 100% marks in JEE

Similar Questions

Trending Articles/News

anam-khan

In JEE Main 2025 April 3 shift 2, chemistry takes U-turn to be tougher than physics, mathematics
anam-khan

JEE Main 2025 April 3 Exam Analysis 2025: Shift 1 paper easy to moderate; chemistry section ‘easiest’
anam-khan

JEE Mains 2025 admit card out for April 7, 8, 9 exams; download on jeemain.nta.nic.in

Latest Question