Browse by Stream
QnA
Home
QnA
Go To Your Study Dashboard

Get Answers to all your Questions

header-bg qa
Filter By
Clear Filter

All Questions

The graph which depicts the results of Rutherford gold foil experiment with \alpha -particles is:  \theta: Scattering angle Y : Number of scattered \alpha - particles detected (Plots are schematic and not to scale)  
Option: 1

Option: 2 

Option: 3 

Option: 4 
 

As Y= N(\theta) \propto \frac{1}{\sin ^{4}\left(\frac{\theta}{2}\right)}

Here when \theta=0 Sin will be zero then Y will tend to infinity.

and that is satisfied by the only option 1

So the correct option is 1.

View Full Answer(1)
Posted by

vishal kumar

The time period of revolution of electron in its ground state orbit in a hydrogen atom is 1.6\times10^{-16}s. The frequency of revolution of the electron in its first excited state (in s-1) is:-
Option: 1  5.6\times 10^{12}
Option: 2 1.6\times 10^{14}
Option: 3 7.8\times 10^{14}  
Option: 4 6.2\times 10^{15}
 

The velocity of the nth orbit

 V_n \ \alpha \ \frac{z}{n}

the radius of the nth orbit

r_n \ \alpha \ \frac{n^2}{z}

 T=\frac{2\pi r}{V}\\ \\ \Rightarrow T \ \propto \ \frac{n^2\times n}{z \times z}=\frac{n^3}{z^2}\\\\ \Rightarrow T \propto\frac{1}{f} \\ \Rightarrow f \ \propto \ \frac{z^2}{n^3}

f_1=\frac{1}{1.6\times 10^{-16}} \ s^{-1}

and 

\frac{f_1}{f_2}=(\frac{n_2}{n_1})^{3}=8\\ \Rightarrow f_2=\frac{f_1}{8}=7.8 \times 10^{14} \ s^{-1}

 

So, option (3) is correct. 

View Full Answer(1)
Posted by

Ritika Jonwal

Crack CUET with india's "Best Teachers"

  • HD Video Lectures
  • Unlimited Mock Tests
  • Faculty Support
cuet_ads

The energy required to ionised a hydrogen like ion in its ground state is 9 Rydbergs. What is the wavelength (in nm) of the radiation emitted when the electron in this ion jumps from the second excited state to the ground state ?
Option: 1 11.4
Option: 2 24.2
Option: 3 8.6
Option: 4 35.8
 

 

 

\frac{hc}{\lambda }=(13.6eV)z^{2}\left [ \frac{1}{n^{2}_{1}}-\frac{1}{n^{2}_{2}} \right ]

n_{1}=1

n_{2}=3

\frac{hc}{\lambda }=(13.6eV)(3^{2})\left [ \frac{1}{1^{2}}-\frac{1}{3^{2}} \right ]

\Rightarrow \frac{hc}{\lambda }=(13.6eV)(9)\left ( \frac{8}{9} \right )

Wavelength=\frac{1240}{8\times 13.6}nm

{\lambda }=11.40nm

Hence the correct option is (1).

View Full Answer(1)
Posted by

avinash.dongre

The first member of the Balmer series of the hydrogen atom has a wavelength of 6561 \AA . The wavelength of the second member of the Balmer series (in nm ) is _______ .
Option: 1 486
Option: 2 972
Option: 3 986
Option: 4 586
 

\begin{aligned} \frac{1}{\lambda} &=\mathrm{R} Z^{2}\left(\frac{1}{n_{1}^{2}}-\frac{1}{n_{2}^{2}}\right) \\ \frac{1}{\lambda_{1}} &=R(1)^{2}\left(\frac{1}{2^{2}}-\frac{1}{3^{2}}\right)=\frac{5 \mathrm{R}}{36} \\ \frac{1}{\lambda_{2}} &=\mathrm{R}(1)^{2}\left(\frac{1}{2^{2}}-\frac{1}{4^{2}}\right)=\frac{3 \mathrm{R}}{16} \\ \frac{\lambda_{2}}{\lambda_{1}} &=\frac{20}{27} \\ \lambda_{2} &=\frac{20}{27} \times 6561 \mathrm{A}=4860 \mathrm{A} \\ &=486 \mathrm{nm} \end{aligned}

View Full Answer(1)
Posted by

vishal kumar

JEE Main high-scoring chapters and topics

Study 40% syllabus and score up to 100% marks in JEE

The activity of a radioactive sample falls from 700 \mathrm{s}^{-1} to  500 \mathrm{s}^{-1} in 30 minutes. Its half life ( in minutes ) is close to :
 
Option: 1 62
Option: 2 72
Option: 3 66
Option: 4 52
 

Activity at any time is given as

A=A_0e^{-\lambda t}\\ \Rightarrow \frac{A}{A_0}=e^{-\lambda t}\\ \Rightarrow ln( \frac{A_0}{A})=\lambda t

After half-life,

 \\t=T_{\frac{1}{2}} \\ A=\frac{A_0}{2}

so \ln 2=\lambda T_{1 / 2}

Now for t=30 \ min \ , A_0=700 \ s^{-1}, \ A=500 \ s^{-1}

\ln \left[\frac{700}{500}\right]=\lambda(30 )

from( (i) and (ii)

\frac{\ln 2}{\ln (7 / 5)}=\frac{T_{1 / 2}}{(30 )}

{t_{1 / 2}} =61.81 \cong 62 \ min

Hence the correct option is (1). 

View Full Answer(1)
Posted by

Ritika Jonwal

A radioactive sample has an average life of 30 ms and is decaying. A capacitor of capacitance 200 \mu F is first charged and later connected with resistor 'R'. If the ratio of charge on capacitor to the activity of radioactive sample is fixed with respect to time then the value of 'R' should be ______\Omega.
 

\begin{gathered} t_{\text {mean }}=30 \times 10^{-3} \mathrm{~S}=\frac{1}{\lambda} \\ C=200 \mu \mathrm{F} \end{gathered}

Let q_{0} be the initial change on the capacitor

\rightarrow q= q_{0}e^{-t/\tau }

Discharging of capacitor condition.

Activity after time  t

A_{t}=A_{0} e^{-\lambda t}

\begin{aligned} &\frac{q_{t}}{A_{t}}=\frac{q_{0}}{A_{0}} \frac{e^{-t / \tau}}{e^{-\lambda t}} \\ &\frac{q_{t}}{A_{t}}=\frac{q_{0}}{A_{0}} e^{(-t / \tau+\lambda t)}=K \end{aligned}

\begin{aligned} \therefore-\frac{t}{\tau} &+\lambda t=0 \\ \frac{1}{\tau} &=\lambda \\ \frac{1}{R C} &=\lambda \end{aligned}

\begin{aligned} t_{\text {mean }}=\frac{1}{\lambda} &=R C \\ \end{aligned}

\begin{aligned} 30 \times 10^{-3}=R \times 200 \times 10^{-6} \\ \end{aligned}

\begin{aligned} R &=\frac{30}{200} \times 10^{+3} \\ \\R &=150 \Omega \end{aligned}

View Full Answer(1)
Posted by

vishal kumar

NEET 2024 Most scoring concepts

    Just Study 32% of the NEET syllabus and Score up to 100% marks

An electron and proton are separated by a large distance. The electron starts approaching the proton with energy 3 \mathrm{eV}. The proton captures the electrons and forms a hydrogen atom in second excited state. The resulting photon is incident on a photosensitive metal of threshold wavelength 4000 \AA. What is the maximum kinetic energy of the emitted photoelectron?
 
Option: 1 7.61 \mathrm{eV}
 
Option: 2 1.41 \mathrm{eV}
Option: 3 3.3 \mathrm{eV}
Option: 4 \text { No photoelectron would be emitted }

Energy lost by electron=Energy of emitted photon

\begin{aligned} &\left(E_{i}-E_{f}\right)=\frac{h c}{\lambda} \\ &\left(3 \operatorname{ev}-\left(\frac{-13.6 \mathrm{eV}}{3^{2}}\right)\right]=\frac{h c}{\lambda} \\ \end{aligned}

\begin{aligned} &{\because \text { for second excitcd state }} \\ &\text { i.e., } For \ (n=3) \Rightarrow E_{n}=\frac{-13.6 \mathrm{eV}}{n^{2}}=\frac{-13.6 \mathrm{eV}}{3^{2}}=-1.51 eV \\ &\frac{h c}{\lambda}=4.51 \mathrm{eV} \end{aligned}

By Einstein's photoelectric equation
\begin{aligned} &\frac{h c}{\lambda}=\phi_{0}+K E_{\max } \\ &4.51 \mathrm{ev}=3.1 \mathrm{ev}+K E_{\text {max }} \\ &K E_{\max }=1.41 \mathrm{eV} \end{aligned}
The correct option is (2)

View Full Answer(1)
Posted by

vishal kumar

Consider the following statements : A. Atoms of each element emit characteristics spectrum. B. According to Bohr's Postulate, an electron in a hydrogen atom, revolves in a certain stationary orbit. C. The density of nuclear matter depends on the size of the nucleus. D. A free neutron is stable but a free proton decay is possible. E. Radioactivity is an indication of the instability of nuclei. Choose the correct answer from the options given below :
Option: 1 A, B, C, D and E
Option: 2 A, B and E only
Option: 3 B and D only
Option: 4 A, C and E only

\rightarrow A free neutron is unstable
\rightarrow Density of nuclear matter is constant for all elements.
The correct option is (2)

View Full Answer(1)
Posted by

vishal kumar

Crack CUET with india's "Best Teachers"

  • HD Video Lectures
  • Unlimited Mock Tests
  • Faculty Support
cuet_ads

The \mathrm{K}_{\alpha} \mathrm{X}-ray  of molybdenum has wavelength 0.071 \mathrm{~nm}. If the energy of a molybdenum atoms with a \mathrm{K} electron knocked out is 27.5 \mathrm{keV}, the energy of this atom when an L electron is knocked out will be ________\mathrm{keV}. (Round off to the nearest integer)
\left[\mathrm{h}=4.14 \times 10^{-15} \mathrm{eVs}, \mathrm{c}=3 \times 10^{8} \mathrm{~ms}^{-1}\right]
 

E_{1} - Energy when an L shell-electron is knocked out
E_{2} - Energy when a K shell-electron is knocked out
E_{2}=27.5 \mathrm{keV}
K_{\alpha} \rightarrowwavelength emitted during knocking out of L-electron
E_{K_{\alpha}} =\frac{1240}{\lambda_{k\alpha}}=\frac{1240\left ( ev \right )}{0.071}
E_{k \alpha }=\frac{1240}{71 \times 10^{-3}}=\frac{1240 \times 10^{3}}{71}(\mathrm{ev})

E_{2}=E_{1}+E_{k_{\alpha}}

27.5 \mathrm{eV}=E_{1}+17.5 \mathrm{keV}

E_{1}=10eV

View Full Answer(1)
Posted by

vishal kumar

A particular hydrogen-like ion emits radiation of frequency 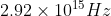2. 92\times 10^{15}Hz when it makes the transition from n = 3 to n = 1, The frequency in Hz of radiation emitted in transition from n =2 to n= 1 will be :
 
Option: 1 6\cdot 57\times 10^{15}
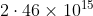
Option: 2 2\cdot 46\times10^{15}
Option: 3 0\cdot 44\times10^{15}
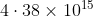
Option: 4 4\cdot 38\times10^{15}

f= 2\cdot 92\times 10^{15}Hz
\Delta E= hf= Rchz^{2}\left [ \frac{1}{n_f^{2}}-\frac{1}{n_i^{2}} \right ]
hf_{1}= Rchz^{2}\left [ \frac{1}{1^{2}}-\frac{1}{3^{2}} \right ]
hf_{2}= Rchz^{2}\left [ \frac{1}{1^{2}}-\frac{1}{2^{2}} \right ]
\frac{f_{1}}{f_{2}}= \frac{\frac{8}{9}}{\frac{3}{4}}= \frac{32}{27}
{f_{2}}= \left ( \frac{27}{32} \right )\times 2\cdot 92\times 10^{15}
{f_{2}}=2\cdot 46\times 10^{15}
The correct option is (2)

View Full Answer(1)
Posted by

vishal kumar

JEE Main high-scoring chapters and topics

Study 40% syllabus and score up to 100% marks in JEE
filter_img