Browse by Stream
QnA
Home

Get Answers to all your Questions

header-bg qa
  • Home
  • Engineering
  • A cylindrical vessel filled with water is released on an inclined surface of angle  as shown in the figu

A cylindrical vessel filled with water is released on an inclined surface of angle \theta as shown in the figure. The friction coefficient of the surface with the vessel is \mu \left ( <\: tan\; \theta \right). Then the constant angle made by the surface of the water with the incline will be:

 

                                            

Option: 1

tan^{-1}\mu


Option: 2

\theta -tan^{-1}\mu


Option: 3

\theta +tan^{-1}\mu


Option: 4

cot^{-1}\mu


Answers (1)

best_answer

As we have learnt in

 

Acceleration of Block sliding down over rough inclined plane -

ma=mg\ sin \theta - F

ma=mg\ sin \theta - \mu R

ma=mg\ sin \theta - \mu mg cos\theta

a=[sin \theta-\mu cos \theta]

 

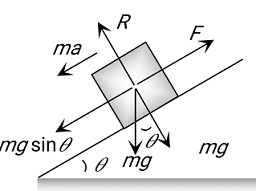
So using the above concept

below is the figure which shows forces acting on a 'particle' on the surface, with respect to vessel.

\left ( mg \; sin\; \theta\; and\; \mu \; mg\; cos\; \theta \ are \ pseudo \ forces \right )

tan\; \phi =\mu\; \; \therefore \phi =tan^{-1}\mu

\phi is angle between normal to the inclined surface and the resultant force. The same angle will be formed between the surface of  water & the inclined surface.

Posted by

vinayak

View full answer

Similar Questions

Latest Question