Go To Your Study Dashboard

Get Answers to all your Questions

header-bg

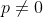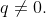The mean and the standard deviation (s.d.) of 10 observations are 20 and 2 respectively. Each of these 10 observations is multiplied by p and then reduced by q, where $p\neq 0$ and $q\neq 0.$ If the new mean and new s.d. become half of their original values, then q is equal to :
Option: 1 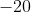$-20$
Option: 2 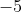$-5$
Option: 3 $10$
Option: 4 $-10$

Answers (1)

best_answer

Some Important Point Regarding Statistics -

Some Important Point Regarding Statistics

The sum of the deviation of an observation from their mean is equal to zero. i.e. $\sum^{n}_{i=1}\left ( x_i-\bar x \right )=0$ .
The sum of the square of the deviation from the mean is minimum, i.e. $\sum^{n}_{i=1}\left ( x_i-\bar x \right )^2\;\text{is least.}$
The mean is affected accordingly if the observations are given a mathematical treatment i.e. addition, subtraction multiplication by a constant term.
If set of n₁ observations has mean $\bar x_1$ and set of n₂ observations has mean $\bar x_2$ , then their combined mean is $\frac{n_1\bar x_1+n_2\bar x_2}{n_1+n_2}$ and the .
If set of n₁ observations has mean $\bar x_1$ and set of n₂ observations has mean $\bar x_2$ then their combined variance is given by

$\sigma ^{2}= \frac{n_{1}\left ( \sigma _{1}^{2}+d_{1}^{2} \right )+n_{2}\left ( \sigma _{2}^{2}+d_{2}^{2} \right )}{n_{1}+n_{2}}$

where, $\\d_1=\bar x_2-\bar x\;\;\;\text{and}\;\;\;d_2=\bar x_ 1-\bar x$

$\\ {\sigma_{1}^{2}=\frac{1}{n_{1}} \sum_{i=1}^{n_{1}}\left(x_{1 i}-\overline{x_{1}}\right)^{2}} \\\\ {\sigma_{2}^{2}=\frac{1}{n_{2}} \sum_{j=1}^{n_{2}}\left(x_{2 j}-\overline{x_{2}}\right)^{2}}$

$\bar x_1,\;\;\bar x_2$ are the means and $\sigma_1,\;\;\sigma_2$ are the standard deviations of two series.

-

$\text{old series} \Rightarrow x_{old}=x_1,x_2,x_3\ldots\ldots x_n$

Old Mean

$\overline x_{old}=\frac{\left (x_1+x_2+x_3\ldots\ldots x_n \right ) }{n}=20$

Old Standard Deviation

$\sigma^{2}_{old}=\frac{1}{n} \sum_{i=1}^{n}\left(x_{i}-\bar{x}\right)^{2}$

If each observation is multiplied with p & then q is subtracted

$\text{new series} \Rightarrow x_{new}=px_1-q,\;px_2-q,\;px_3-q\ldots\ldots \;px_n-q$

New mean is the half of old mean

$\\ \overline x_{new}=\frac{p\left (x_1+x_2+x_3\ldots\ldots x_n \right )-nq}{n} =10\\\\ \overline x_{new}=p\;\overline x_{old}-q=10\\\\ \overline x_{new}=20p-q=10\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\ldots(i)$

and new standard deviations is the half of old mean

$\sigma^{2}_{new}=\frac{1}{n} \sum_{i=1}^{n}\left(x_{new}-\bar{x}_{new}\right)^{2}$

$\sigma^{2}_{new}=\frac{1}{n} \sum_{i=1}^{n}\left[(px_{i}-q)-(p\bar{x}_{old}-q)\right]^{2}$

$\sigma^{2}_{new}=\frac{1}{n} \sum_{i=1}^{n}\left(px_{i}-p\bar{x}_{old}\right)^{2}$

$\sigma^{2}_{new}=\frac{1}{n}p^2 \sum_{i=1}^{n}\left(x_{i}-\bar{x}_{old}\right)^{2}$

$\sigma_{new}=|p|\sigma_{old}$

$1=2|p|$

$p=\pm0.5$

Case 1 $p=0.5$

Using equation 1

we get

$\\20p-q=10\\10-q=10\\q=0$

Case 1 $p=-0.5$

Using equation 1

we get

$\\20p-q=10\\-10-q=10\\q=-20$

Correct Option (1)

Posted by

Kuldeep Maurya

View full answer

JEE Main high-scoring chapters and topics

Study 40% syllabus and score up to 100% marks in JEE

Similar Questions

Trending Articles/News

anam-khan

JEE Main 2025 registration ends tomorrow; changes introduced in exam this year

anam-khan

BTech Admission 2025 Live: JEE Mains registrations closing tomorrow; VITEEE, MET exam dates out

anam-khan

JEE Main 2025 Live: NTA to close JEE registration on Friday; apply at jeemain.nta.nic.in, correction window

Latest Question