Browse by Stream
QnA
Home
QnA
Go To Your Study Dashboard

Get Answers to all your Questions

header-bg qa
  • Home
  • Engineering
  • The ratio of velocities of a planet at perigee and apogee   is equal to -<div cl

The ratio of velocities of a planet at perigee and apogee \frac{v_p}{v_a}  is equal to -

Option: 1

1


Option: 2

\frac{1-e}{1+e}


Option: 3

\frac{1+e}{1-e}


Option: 4

\frac{1+e^2}{1-e^2}


Answers (1)

best_answer

As we have learnt,

 

Velocity of planet in terms of Eccentricity -

V_{a}=\sqrt{\frac{GM}{a}\left ( \frac{1-e}{1+e} \right )}

V_{p}=\sqrt{\frac{GM}{a}\left ( \frac{1+e}{1-e} \right )}

V_{A}= Velocity of planet at apogee

V_{p}= Velocity of perigee

- wherein

Eccentricity (e) = \frac{c}{a}

r_{p}=a-c

r_{a}=a+c

 

 

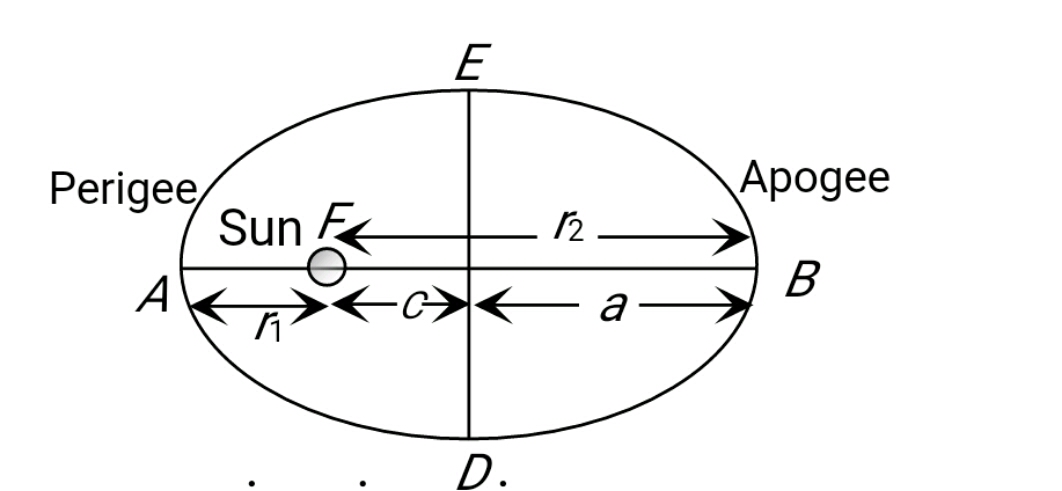
By conservation of angular momentum,

mv_pr_p = mv_ar_a

\Rightarrow \frac{v_p}{v_a} = \frac{r_a}{r_p} = \frac{a+c}{a-c} = \frac{1+e}{1-e} 

Posted by

manish

View full answer

Similar Questions

Latest Question