Go To Your Study Dashboard

Get Answers to all your Questions

header-bg

What is the minimum energy required to launch a satellite of mass m from the surface of a planet of mass M and radius R in a circular orbit at an altitude of 2R ?

Option: 1
$\frac{GmM}{3R}$

Option: 2
$\frac{5GmM}{6R}$

Option: 3
$\frac{2GmM}{3R}$

Option: 4
$\frac{GmM}{2R}$

Answers (1)

best_answer

Work done in changing the orbit -

$W=E_{2}-E_{1}$

$W=\frac{GMm}{2}\left [ \frac{1}{r_{1}}-\frac{1}{r_{2}} \right ]$

$W\rightarrow work\: done$

$r_{1}\rightarrow radius\: of\: 1st\: orbit$

$r_{2}\rightarrow radius\: of\: 2nd\: orbit$

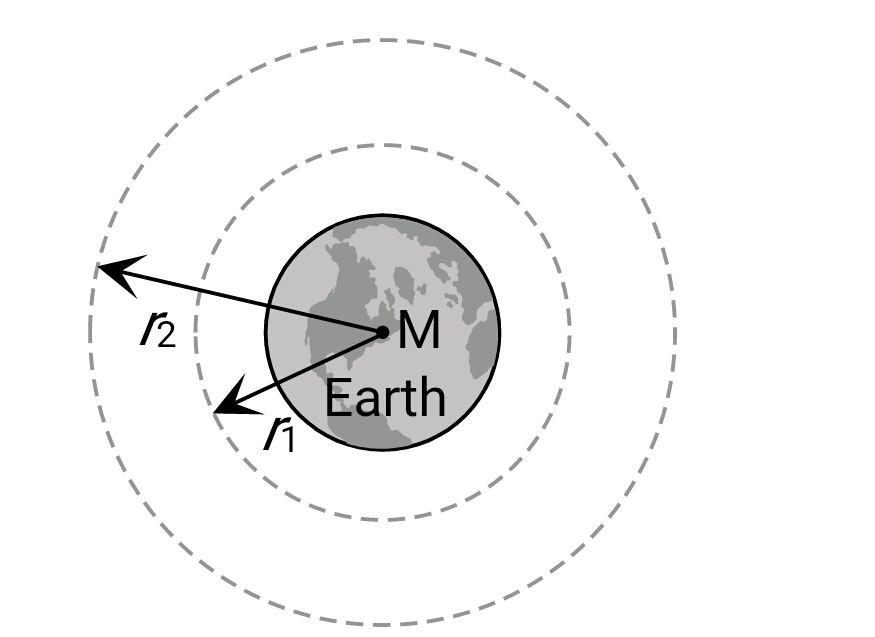
- wherein

When satellite is transferred to higher orbit

$\left ( r_{2}>r_{1} \right )$

Use given equation

Let k.i= 0 at surface and we providing minimum energy $E_{min}$ to launch satellite

So

$E_{i} =E_{min}+ k.i +p.i =E_{min}+ 0 + \frac{-GMm}{R} = E_{min}-\frac{GMm}{R}$

the velocity of the satellite a distance 2 R from the surface of the planet total oneggy of the satellite.

$E_{f} = \frac{1}{2} mv^2 - \frac{GMm}{\left ( R+2R \right )}$

$E_{f} = \frac{1}{2}m (\sqrt{\frac{GM}{R+2R}})^{2}- \frac{GMm}{3R}$

$= \frac{1}{2} \frac{Gmm}{3R} - \frac{Gmm}{3R} = -\frac{Gmm}{6R}$

$\therefore$ minnimum energy required to launch setelite

Apply energy conservation

So $E_{f} =E_{i}$

$E_{min} = E_{f} -E _{i} = \frac{-GMm}{6R} -( \frac{-GMm}{R})$

$= \frac{-GMm}{6R} +\frac{GMm}{R}$

$= \frac{5GMm}{6R}$

$Ans \left ( 2 \right )$

Posted by

HARSH KANKARIA

View full answer

Similar Questions

Latest Question