Browse by Stream
QnA
Home
QnA
Go To Your Study Dashboard

Get Answers to all your Questions

header-bg qa
  • Home
  • Medical
  • A train of mass M is moving on a circular track of radius ' R ' with constant speed V. The length of the train is half of the perimeter of the track. The linear momentum of the tr

A train of mass M is moving on a circular track of radius ' R ' with constant speed V. The length of the train is half of the perimeter of the track. The linear momentum of the train will be

Option: 1

0


Option: 2

\frac{2MV}{\pi}


Option: 3

MVR


Option: 4

MV


Answers (1)

best_answer

 

Centre of Mass of semicircular ring -

It lies at a distance of \frac{2R}{\pi} from centre of the ring along its axis.

-

 

 

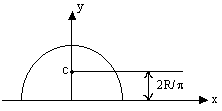
 If we treat the train as a ring of mass 'M' then its COM will be at a distance \frac{2R}{\pi}  from the centre of the circle. Velocity of centre of mass is :

V_{CM}= R_{CM}.\omega = \frac{2R}{\pi }.\omega = \frac{2R}{\pi}\left ( \frac{V}{R} \right )         \left ( \because \omega = \frac{V}{R} \right )

\Rightarrow V_{CM}= \frac{2V}{\pi} \Rightarrow MV_{CM} = \frac{2MV}{\pi}

As the linear momentum of any system = MVCM

 \therefore The linear momentum of the train   = \frac{2MV}{\pi}

Posted by

chirag

View full answer

NEET 2024 Most scoring concepts

    Just Study 32% of the NEET syllabus and Score up to 100% marks

Similar Questions

Trending Articles/News

anam-khan

NEET UG: Centre assures SC it will implement recommendations of expert panel on NTA exams
anam-khan

NEET fiasco, paper leaks, Kolkata rape case; student movements, exam controversies that rocked 2024
anam-khan

NTA notifies NEET UG 2025 syllabus, new official website is neet.nta.nic.in

Latest Question