-
Engineering and Architecture
Exams
Colleges
Predictors
Resources
-
Computer Application and IT
Quick Links
Colleges
-
Pharmacy
Colleges
Resources
-
Hospitality and Tourism
Colleges
Resources
Diploma Colleges
-
Competition
Other Exams
Resources
-
School
Exams
Top Schools
Products & Resources
-
Study Abroad
Top Countries
Resources
-
Arts, Commerce & Sciences
Exams
Colleges
Upcoming Events
Resources
-
Management and Business Administration
Colleges & Courses
Predictors
-
Learn
Law Preparation
MBA Preparation
Engineering Preparation
Medical Preparation
-
Online Courses and Certifications
Top Streams
Specializations
- Digital Marketing Certification Courses
- Cyber Security Certification Courses
- Artificial Intelligence Certification Courses
- Business Analytics Certification Courses
- Data Science Certification Courses
- Cloud Computing Certification Courses
- Machine Learning Certification Courses
- View All Certification Courses
Resources
-
Medicine and Allied Sciences
Colleges
Predictors
Resources
-
Law
Resources
Colleges
-
Animation and Design
Exams
Predictors & Articles
Colleges
Resources
-
Media, Mass Communication and Journalism
Colleges
Resources
-
Finance & Accounts
Top Courses & Careers
Colleges
Get Answers to all your Questions


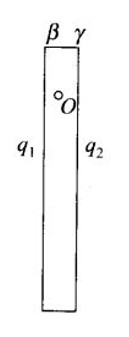
Two fixed, identical conducting plates , each of surface area S are charged to –Q and q, respectively, where
. A third identical plate (
), free to move is located on the other side of the plate with charge q at a distance d (Fig 1.13). The third plate is released and collides with the plate β. Assume the collision is elastic and the time of collision is sufficient to redistribute charge amongst

(a) Find the electric field acting on the plate before the collision.
(b) Find the charges on β and after the collision.
(c) Find the velocity of the plate after the collision and at a distance d from the plate β.
Answers (1)
Explanation:- Here, is the proper solution
(a) Net electric field field at plate before collision is vector sum of electric field at plate
due to plate
.
The electric field at plate due to plate
is
The electric field at plate due to plate
is
Hence the net electric field at plate before collision is
Or to the left, if
(b) During collision, plates are in contact with each other, hence their potential becomes the same.
Suppose charge on plate and charge on plate
. at any point O , in between the two plates, the electric field must be zero.
Electric field at O due to ,
Electric field at O due to ,
Electric field at O due to ,
As the electric field at O is zero, therefore
As there is no loss of charge on collision,
On solving Equ. (1) and (2) We get
Charge on plate
Charge on plate
(c) Let the velocity be at the distance d from plate
after the collision.
If m is the mass of the plate , then the gain in K.E. over the round trip must be equal to the work done by the electric field.
After the collision, electric field at plate is
Just before collision, electric field at plate is
If F1 is force on plate before collision., then
Total work done by the electric field is the round trip movement of plate ,
If m is the mass of plate , the KE gained by the plate =
According to work energy principle,
Similar Questions
- 100 g of liquid A (molar mass 140 g mol^-1) was dissolved in 1000 g of liquid B (molar mass 180 g mol^-1).The vapour pressure of pure liquid B was found to be 500 torr.
- 100 turn rectangular coil ABCD (in X-Y plane) is hung from one arm of a balance (shown in figure). A mass 500 g is added to the other arm to balance the weight of the coil.
- 100m long antenna is mounted on a 500m tall building The complex can become a transmission tower for waves with λ (a) ~ 400 m (b) ~ 25 m (c) ~ 150 m (d) ~ 2400 m

